Hostname: page-component-78c5997874-xbtfd Total loading time: 0 Render date: 2024-11-10T16:07:29.916Z Has data issue: false hasContentIssue false
  • English
  • Français

On bucket increasing trees, clustered increasing trees and increasing diamonds

Part of: Limit theorems Graph theory

Published online by Cambridge University Press:  13 October 2021

Markus Kuba  and
Alois Panholzer
Markus Kuba*
Affiliation:
Department Applied Mathematics and Physics, University of Applied Sciences - Technikum Wien, Höchstädtplatz 5, 1200 Wien, Austria
Alois Panholzer
Affiliation:
Institut für Diskrete Mathematik und Geometrie, Technische Universität Wien, Wiedner Hauptstr. 8-10/104, 1040 Wien, Austria
*
*Corresponding author. Email: kuba@technikum-wien.at
Get access Rights & Permissions [Opens in a new window]

Abstract

In this work we analyse bucket increasing tree families. We introduce two simple stochastic growth processes, generating random bucket increasing trees of size n, complementing the earlier result of Mahmoud and Smythe (1995, Theoret. Comput. Sci.144 221–249.) for bucket recursive trees. On the combinatorial side, we define multilabelled generalisations of the tree families d-ary increasing trees and generalised plane-oriented recursive trees. Additionally, we introduce a clustering process for ordinary increasing trees and relate it to bucket increasing trees. We discuss in detail the bucket size two and present a bijection between such bucket increasing tree families and certain families of graphs called increasing diamonds, providing an explanation for phenomena observed by Bodini et al. (2016, Lect. Notes Comput. Sci.9644 207–219.). Concerning structural properties of bucket increasing trees, we analyse the tree parameter 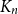$K_n$ . It counts the initial bucket size of the node containing label n in a tree of size n and is closely related to the distribution of node types. Additionally, we analyse the parameters descendants of label j and degree of the bucket containing label j, providing distributional decompositions, complementing and extending earlier results (Kuba and Panholzer (2010), Theoret. Comput. Sci.411(34–36) 3255–3273.).

Keywords

Bucket increasing treesclustered treesstochastic growth processesdescendantsnode-degreeslimiting distributions

MSC classification

Primary: 05C05: Trees
Secondary: 60F05: Central limit and other weak theorems
Type
Paper
Information
Combinatorics, Probability and Computing , Volume 31 , Issue 4 , July 2022 , pp. 629 - 661
Copyright
© The Author(s), 2021. Published by Cambridge University Press

Access options

Get access to the full version of this content by using one of the access options below. (Log in options will check for institutional or personal access. Content may require purchase if you do not have access.)

References

Berger, N., Borgs, C., Chayes, J. T., D’Souza, R. M. and Kleinberg, R. D. (2004) Competition-induced preferential attachment. In Proceedings of the 31st International Colloquium on Automata, Languages and Programming (ICALP), Vol. 3142 of Lecture Notes in Computer Science, pp. 208–221.CrossRefGoogle Scholar
Berger, N., Borgs, C., Chayes, J. T., D’Souza, R. M. and Kleinberg, R. D. (2005) Degree distribution of competition-induced preferential attachment graphs. Comb. Prob. Comput. 14 697–721.CrossRefGoogle Scholar
Bergeron, F., Flajolet, P. and Salvy, B. (1992) Varieties of increasing trees. Lect. Notes Comput. Sci. 581 24–48.CrossRefGoogle Scholar
Bodini, O., Dien, M., Fontaine, X., Genitrini, A. and Hwang, H.-K. (2016) Increasing diamonds. Lect. Notes Comput. Sci. 9644 207–219.CrossRefGoogle Scholar
Drmota, M. (2009) Random Trees. Springer.CrossRefGoogle Scholar
Flajolet, P. and Segdewick, R. (2009) Analytic Combinatorics. Cambridge University Press.CrossRefGoogle Scholar
Janson, S. (2004) Functional limit theorems for multitype branching processes and generalized Pólya urns. Stochast. Process. Appl. 110 177–245.CrossRefGoogle Scholar
Janson, S., Kuba, M. and Panholzer, A. (2011) Generalized Stirling permutations, families of increasing trees and URN models. J. Comb. Theory Ser. A 118 94–114.CrossRefGoogle Scholar
Kazemi, R. (2014) Depth in bucket recursive trees with variable capacities of buckets. Acta Mathematica Sinica, English Series 30 305–310.CrossRefGoogle Scholar
Kuba, M. and Panholzer, A. (in preparation) Characterization of growth processes generating bucket increasing trees.Google Scholar
Kuba, M. and Panholzer, A. (2007) On the degree distribution of the nodes in increasing trees. J. Comb. Theory Ser. A 114 597–618.CrossRefGoogle Scholar
Kuba, M. and Panholzer, A. (2009) A combinatorial approach for analyzing the number of descendants in increasing trees and related parameters. Quaestiones Mathematicae 32 91–114.CrossRefGoogle Scholar
Kuba, M. and Panholzer, A. (2010) A combinatorial approach to the analysis of bucket recursive trees and variants. Theoret. Comput. Sci. 411(34–36) 3255–3273.CrossRefGoogle Scholar
Kuba, M. and Panholzer, A. (2012) Bilabelled increasing trees and hook-length formulas. Eur. J. Comb. 33 248–258.CrossRefGoogle Scholar
Kuba, M. and Panholzer, A. (2016) Combinatorial families of multilabelled increasing trees and hook-length formulas. Discrete Math. 339 227–254.CrossRefGoogle Scholar
Kuba, M. and Panholzer, A. (2016) Moment sequences and mixed Poisson distributions. Prob. Surv. 13 89–155.CrossRefGoogle Scholar
Kuba, M. and Panholzer, A. (2019) Combinatorial analysis of growth models for series-parallel networks. Comb. Prob. Comput. 28 574–599.CrossRefGoogle Scholar
Mahmoud, H. and Smythe, R. (1995) Probabilistic analysis of bucket recursive trees. Theoret. Comput. Sci. 144 221–249.CrossRefGoogle Scholar
Mahmoud, H. and Smythe, R. (1995) A survey of recursive trees. Theoret. Prob. Math. Stat. 51 1–37.Google Scholar
Müller, N. S. (2019) Central limit theorem analogues for multicolour urn models. submitted.Google Scholar
Panholzer, A. and Prodinger, H. (2007) The level of nodes in increasing trees revisited. Random Struct. Alg. 31 203–226.CrossRefGoogle Scholar
Pittel, B. (1994) Note on the heights of random recursive trees and random m-ary search trees. Random Struct. Alg. 5 337–347.CrossRefGoogle Scholar
Prodinger, H. (1996) Depth and path length of heap ordered trees. Int. J. Found. Comput. Sci. 7 293–299.CrossRefGoogle Scholar
Prodinger, H. (1996) Descendants in heap ordered trees - or - a triumph of computer algebra. Electron. J. Comb. 3(# R29).CrossRefGoogle Scholar