We consider two sequences $a(n)$ and $b(n)$, $1\leq n<\infty $, generated by Dirichlet series

$$ \begin{align*}\sum_{n=1}^{\infty}\frac{a(n)}{\lambda_n^{s}}\qquad\text{and}\qquad \sum_{n=1}^{\infty}\frac{b(n)}{\mu_n^{s}},\end{align*} $$

satisfying a familiar functional equation involving the gamma function $\Gamma (s)$. Two general identities are established. The first involves the modified Bessel function $K_{\mu }(z)$, and can be thought of as a ‘modular’ or ‘theta’ relation wherein modified Bessel functions, instead of exponential functions, appear. Appearing in the second identity are $K_{\mu }(z)$, the Bessel functions of imaginary argument $I_{\mu }(z)$, and ordinary hypergeometric functions ${_2F_1}(a,b;c;z)$. Although certain special cases appear in the literature, the general identities are new. The arithmetical functions appearing in the identities include Ramanujan’s arithmetical function $\tau (n)$, the number of representations of n as a sum of k squares $r_k(n)$, and primitive Dirichlet characters $\chi (n)$.

Let $(X,+)$ be an Abelian group and $E$ be a Banach space. Suppose that $f:X\rightarrow E$ is a surjective map satisfying the inequality

$$\begin{eqnarray}|\,\Vert f(x)-f(y)\Vert -\Vert f(x-y)\Vert \,|\leq {\it\varepsilon}\min \{\Vert f(x)-f(y)\Vert ^{p},\Vert f(x-y)\Vert ^{p}\}\end{eqnarray}$$

${\it\varepsilon}>0$

$p>1$

$x,y\in X$

$f$

$0<p\leq 1$

$f$

$E$

$F$

${\it\epsilon}>0$

$p>1$

$$\begin{eqnarray}|\,\Vert f(x)-f(y)\Vert -\Vert f(u)-f(v)\Vert \,|\leq {\it\epsilon}\min \{\Vert f(x)-f(y)\Vert ^{p},\Vert f(u)-f(v)\Vert ^{p}\}\end{eqnarray}$$

$\Vert x-y\Vert =\Vert u-v\Vert$

$f$

$\dim E\geq 2$

$K\neq 0$

$Kf$

By exploring the relations among functional equations, harmonic analysis and representation theory, we give a unified and very accessible approach to solve three important functional equations — the d'Alembert equation, the Wilson equation, and the d'Alembert long equation — on compact groups.

In this paper, we prove that a non–zero power series $F(z\text{)}\in \mathbb{C}\text{ }[[z]]$ satisfying

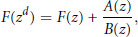$$F({{z}^{d}})\,=\,F(z)\,+\,\frac{A(z)}{B(z)},$$

where 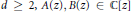$d\,\ge \,2,\,A(z),\,B(z)\,\in \,\mathbb{C}[z]$, with $A(z)\,\ne \,0$ and 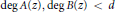$\deg \,A(z),\,\deg \,B(z)\,<\,d$ is transcendental over 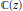$\mathbb{C}(z)$. Using this result and a theorem of Mahler’s, we extend results of Golomb and Schwarz on transcendental values of certain power series. In particular, we prove that for all 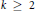$k\,\ge \,2$ the series 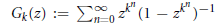${{G}_{k}}(z):=\mathop{\sum }_{n=0}^{\infty }{{z}^{{{k}^{n}}}}{{(1-{{z}^{{{k}^{n}}}})}^{-1}}$ is transcendental for all algebraic numbers $z$ with 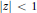$\left| z \right|\,<\,1$. We give a similar result for 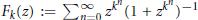${{F}_{k}}(z):=\mathop{\sum }_{n=0}^{\infty }{{z}^{{{k}^{n}}}}{{(1+{{z}^{{{k}^{n}}}})}^{-1}}$. These results were known to Mahler, though our proofs of the function transcendence are new and elementary; no linear algebra or differential calculus is used.

A system of functional equations relating the Euler characteristics of moduli spaces of stable representations of quivers and the Euler characteristics of (Hilbert-scheme-type) framed versions of quiver moduli is derived. This is applied to wall-crossing formulas for the Donaldson–Thomas type invariants of M. Kontsevich and Y. Soibelman, in particular confirming their integrality.

This paper is devoted to the study of certain zeta distributions associated with simple non-Euclidean Jordan algebras. An explicit form of the corresponding functional equation and Bernstein-type identities is obtained.

Let $G$ be a compact group.

Let $\sigma$ be a continuous involution of $G$. In this paper, we are concerned by the following functional equation

$$\int\limits_{G}{f\left( xty{{t}^{-1}} \right)dt}\,+\int\limits_{G}{f\left( xt\sigma \left( y \right){{t}^{-1}} \right)dt}=2g\left( x \right)h\left( y \right),\,\,\,x,y\in G,$$

where $f,g,h:G\mapsto \mathbb{C}$, to be determined, are complex continuous functions on $G$ such that $f$ is central. This equation generalizes d’Alembert's and Wilson's functional equations. We show that the solutions are expressed by means of characters of irreducible, continuous and unitary representations of the group $G$.

We investigate a connection between the differential of polylogarithms (as considered by Cathelineau) and a finite variant of them. This allows to answer a question raised by Kontsevich concerning the construction of functional equations for the finite analogs, using in part the p-adic version of polylogarithms and recent work of Besser. Kontsevich's original unpublished note is supplied (with his kind permission) in an ‘Appendix’ at the end of the paper.

The finite nth polylogarithm lin(z) ∈ Z/p(z) is defined as [sum ]k=1p−1zk/kn. We state and prove the following theorem. Let Lik: $ C$p → $ C$p be the p-adic polylogarithms defined by Coleman. Then a certain linear combination Fn of products of polylogarithms and logarithms, with coefficients which are independent of p, has the property that p1−nDFn(z) reduces modulo p>n+1 to lin−1(σ(z)), where D is the Cathelineau operator z(1−z)d/dz and σ is the inverse of the p-power map. A slightly modified version of this theorem was conjectured by Kontsevich. This theorem is used by Elbaz-Vincent and Gangl to deduce functional equations of finite polylogarithms from those of complex polylogarithms.

In this short communication, some of the recent results of Liu (1998) and Biggins and Kyprianou (1997), concerning solutions to a certain functional equation associated with the branching random walk, are strengthened. Their importance is emphasized in the context of travelling wave solutions to a discrete version of the KPP equation and the connection with the behaviour of the rightmost particle in the nth generation.

Let an interval I ⊂ ℝ and subsets D0, D1 ⊂ I with D0 ∪ D1 = I and D0 ∩ D1 = Ø be given, as well as functions r0: D0 → I, r1: D1 → I. We investigate the system (S) of two functional equations for an unknown function f: I → [0, 1]: We derive conditions for the existence, continuity and monotonicity of a solution. It turns out that the binary expansion of a solution can be computed in a simple recursive way. This recursion is algebraic for, e.g., inverse trigonometric functions, but also for the elliptic integral of the first kind. Moreover, we use (S) to construct two kinds of peculiar functions: surjective functions whose intervals of constancy are residual in I, and strictly increasing functions whose derivative is 0 almost everywhere.

Let W be the usual almost-sure limit random variable in a supercritical simple branching process; we study its tail behaviour. For the left tail, we distinguish two cases, the ‘Schröder' and ‘Böttcher' cases; both appear in work of Harris and Dubuc. The Schröder case is related to work of Karlin and McGregor on embeddability in continuous-time (Markov) branching processes. New results are obtained for the Böttcher case; there are links with recent work of Barlow and Perkins on Brownian motion on a fractal. The right tail is also considered. Use is made of recent progress in Tauberian theory.

If {Zn} is a Galton–Watson branching process with infinite mean, it is shown that under certain conditions there exist constants {cn} and a function L, slowly varying at 0, such that converges almost surely to a non-degenerate random variable, whose distribution function satisfies a certain functional equation. The method is then extended to a continuous-time Markov branching process {Zt} with infinite mean, where it is shown that there is always a function φ, slowly varying at 0, such that converges almost surely to a non-degenerate random variable, and a necessary and sufficient condition is given for this convergence to be equivalent to convergence of for some constant α > 0.

If a subcritical Galton-Watson process is initiated with an arbitrary mass distribution, then it is known that under certain conditions proper conditional limit distributions exist, depending on a single parameter. It is shown here that there is a one-to-one correspondence between these distributions and those arising from the process with a linear offspring probability generating function.

The paper considers some properties of the reversible counters system of Lampard [1]. Lampard considers the properties of the process imbedded at the epochs when the counter receiving pulses from the ‘down’ process reaches zero. We consider here the time-dependent and stationary distributions of the original process. Their derivation gives rise to some interesting functional equations, besides being useful for studying queues with Markov-dependent inter-arrival or service times.

The multitype Galton-Watson process is considered both with and without immigration. Proofs are given for the existence of invariant measures and their uniqueness is examined by functional equation methods. Theorem 2.1 proves the uniqueness, under certain conditions, of solutions of a multidimensional Schröder equation. Regular variation is shown to play a role in the multitype theory.

For the general age-dependent branching process subject to an arbitrary pattern of immigration asymptotic distributions are studied in the supercritical and critical cases. In the supercritical case a new functional form is given for the limiting cumulant-generating function and in the critical case moment conditions are given under which a randomized gamma limit law prevails. The age-structure is also considered.