No CrossRef data available.
Article contents
On the Generalized d’Alembert's and Wilson's Functional Equations on a Compact Group
Published online by Cambridge University Press: 20 November 2018
Abstract
Let 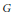 $G$ be a compact group.
$G$ be a compact group.
Let  $\sigma$ be a continuous involution of
$\sigma$ be a continuous involution of 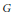 $G$ . In this paper, we are concerned by the following functional equation
$G$ . In this paper, we are concerned by the following functional equation
 $$\int\limits_{G}{f\left( xty{{t}^{-1}} \right)dt}\,+\int\limits_{G}{f\left( xt\sigma \left( y \right){{t}^{-1}} \right)dt}=2g\left( x \right)h\left( y \right),\,\,\,x,y\in G,$$
$$\int\limits_{G}{f\left( xty{{t}^{-1}} \right)dt}\,+\int\limits_{G}{f\left( xt\sigma \left( y \right){{t}^{-1}} \right)dt}=2g\left( x \right)h\left( y \right),\,\,\,x,y\in G,$$
where 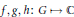 $f,g,h:G\mapsto \mathbb{C}$ , to be determined, are complex continuous functions on
$f,g,h:G\mapsto \mathbb{C}$ , to be determined, are complex continuous functions on 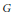 $G$ such that
$G$ such that 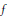 $f$ is central. This equation generalizes d’Alembert's and Wilson's functional equations. We show that the solutions are expressed by means of characters of irreducible, continuous and unitary representations of the group
$f$ is central. This equation generalizes d’Alembert's and Wilson's functional equations. We show that the solutions are expressed by means of characters of irreducible, continuous and unitary representations of the group 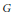 $G$ .
$G$ .
Keywords
Information
- Type
- Research Article
- Information
- Copyright
- Copyright © Canadian Mathematical Society 2005

