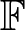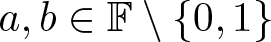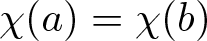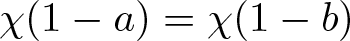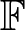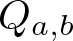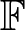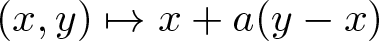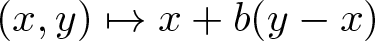Let $\mathbb F$ be a finite field of odd order and $a,b\in\mathbb F\setminus\{0,1\}$ be such that $\chi(a) = \chi(b)$ and $\chi(1-a)=\chi(1-b)$, where χ is the extended quadratic character on $\mathbb F$. Let $Q_{a,b}$ be the quasigroup over $\mathbb F$ defined by $(x,y)\mapsto x+a(y-x)$ if $\chi(y-x) \geqslant 0$, and $(x,y)\mapsto x+b(y-x)$ if 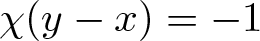$\chi(y-x) = -1$. We show that $Q_{a,b} \cong Q_{c,d}$ if and only if $\{a,b\}= \{\alpha(c),\alpha(d)\}$ for some 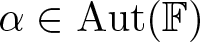$\alpha\in \operatorname{Aut}(\mathbb F)$. We also characterize $\operatorname{Aut}(Q_{a,b})$ and exhibit further properties, including establishing when 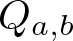$Q_{a,b}$ is a Steiner quasigroup or is commutative, entropic, left or right distributive, flexible or semisymmetric. In proving our results, we also characterize the minimal subquasigroups of 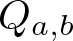$Q_{a,b}$.

Let q be an odd prime power and suppose that $a,b\in \mathbb {F}_q$ are such that $ab$ and $(1{-}a)(1{-}b)$ are nonzero squares. Let $Q_{a,b} = (\mathbb {F}_q,*)$ be the quasigroup in which the operation is defined by $u*v=u+a(v{-}u)$ if $v-u$ is a square, and $u*v=u+b(v{-}u)$ if $v-u$ is a nonsquare. This quasigroup is called maximally nonassociative if it satisfies $x*(y*z) = (x*y)*z \Leftrightarrow x=y=z$. Denote by $\sigma (q)$ the number of $(a,b)$ for which $Q_{a,b}$ is maximally nonassociative. We show that there exist constants $\alpha \approx 0.029\,08$ and $\beta \approx 0.012\,59$ such that if $q\equiv 1 \bmod 4$, then $\lim \sigma (q)/q^2 = \alpha $, and if $q \equiv 3 \bmod 4$, then $\lim \sigma (q)/q^2 = \beta $.