A Martin-Löf random sequence is an infinite binary sequence with the property that every initial segment σ has prefix-free Kolmogorov complexity K(σ) at least ∣σ∣ − c, for some constant c ϵ ω. Informally, initial segments of Martin-Löf randoms are highly complex in the sense that they are not compressible by more than a constant number of bits. However, all Martin-Löf randoms necessarily have contiguous substrings of arbitrarily low complexity. If we demand that all substrings of a sequence be uniformly complex, then we arrive at the notion of shift-complex sequences. In this paper, we collect some of the existing results on these sequences and contribute two new ones. Rumyantsev showed that the measure of oracles that compute shift-complex sequences is zero. We strengthen this result by proving that the Martin-Löf random sequences that do not compute shift-complex sequences are exactly the incomplete ones, in other words, the ones that do not compute the halting problem. In order to do so, we make use of the characterization by Franklin and Ng of the class of incomplete Martin-Löf randoms via a notion of randomness called difference randomness. Turning to the power of shift-complex sequences as oracles, we show that there are shift-complex sequences that do not compute Martin-Löf random (or even Kurtz random) sequences.
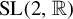 ${\mathrm {SL}}(2,{\mathbb R})$ cocycles and applications to Schrödinger operators defined over Boshernitzan subshifts
${\mathrm {SL}}(2,{\mathbb R})$ cocycles and applications to Schrödinger operators defined over Boshernitzan subshifts
 ${C}^{\ast } $-ALGEBRAS ASSOCIATED WITH LAMBDA-SYNCHRONIZING SUBSHIFTS AND FLOW EQUIVALENCE
${C}^{\ast } $-ALGEBRAS ASSOCIATED WITH LAMBDA-SYNCHRONIZING SUBSHIFTS AND FLOW EQUIVALENCE