Skip to main content Accessibility help

We use cookies to distinguish you from other users and to provide you with a better experience on our websites. Close this message to accept cookies or find out how to manage your cookie settings.

Close cookie message
×
Hostname: page-component-cd9895bd7-dk4vv Total loading time: 0 Render date: 2024-12-28T02:04:29.458Z Has data issue: false hasContentIssue false

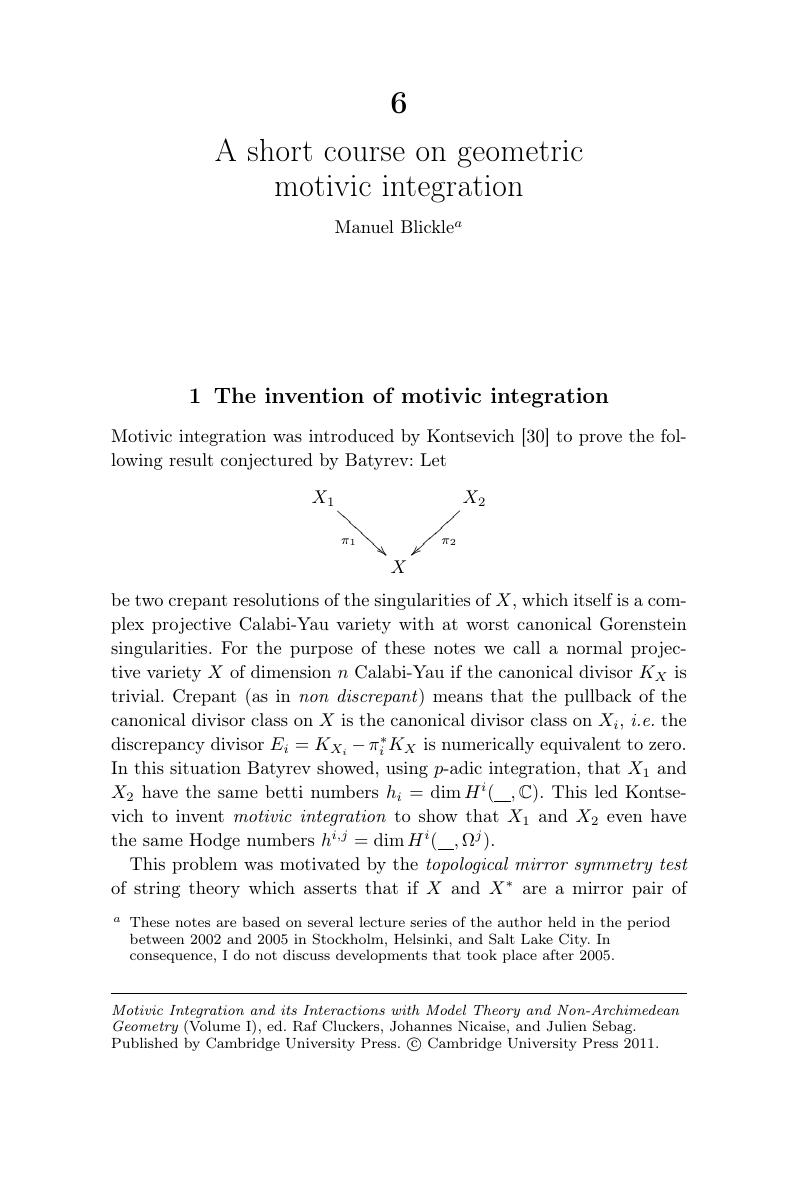
6 - A short course on geometric motivic integration

Published online by Cambridge University Press:  07 October 2011

By
Manuel Blickle
Edited by
Raf Cluckers ,
Johannes Nicaise  and
Julien Sebag
Manuel Blickle
Affiliation:
Johannes Gutenberg-Universität Mainz
Raf Cluckers
Affiliation:
Université de Lille
Johannes Nicaise
Affiliation:
Katholieke Universiteit Leuven, Belgium
Julien Sebag
Affiliation:
Université de Rennes I, France
Get access

Summary

A summary is not available for this content so a preview has been provided. Please use the Get access link above for information on how to access this content.
Image of the first page of this content. For PDF version, please use the ‘Save PDF’ preceeding this image.'
Type
Chapter
Information
Motivic Integration and its Interactions with Model Theory and Non-Archimedean Geometry , pp. 189 - 243
Publisher: Cambridge University Press
Print publication year: 2011

Access options

Get access to the full version of this content by using one of the access options below. (Log in options will check for institutional or personal access. Content may require purchase if you do not have access.)

References

[1] Dan, Abramovich, Kalle, Karu, Kenji, Matsuki, and Jarosław, Włodarczyk, Torification and factorization of birational maps, J. Amer. Math. Soc. 15 (2002), no. 3, 531–572 (electronic).Google Scholar
[2] Victor V., Batyrev, Stringy Hodge numbers of varieties with Goren-stein canonical singularities, Integrable systems and algebraic geometry (Kobe/Kyoto, 1997), World Sci. Publishing, River Edge, NJ, 1998, pp. 1–32.Google Scholar
[3] Victor V., Batyrev and Lev A., Borisov, Mirror duality and string-theoretic Hodge numbers, Invent. Math. 126 (1996), no. 1, 183–203.Google Scholar
[4] Franziska, Bittner, The universal Euler characteristic for varieties of characteristic zero, Compos. Math. 140 (2004), no. 4, 1011–1032.Google Scholar
[5] Siegfried, Bosch, Werner, Lütkebohmert, and Michel, Raynaud, Néron models, Ergebnisse der Mathematik und ihrer Grenzgebiete (3), vol. 21, Springer-Verlag, Berlin, 1990.Google Scholar
[6] Alastair, Craw, An introduction to motivic integration, Strings and geometry, Clay Math. Proc., vol. 3, Amer. Math. Soc., Providence, RI, 2004, pp. 203–225.Google Scholar
[7] Pierre, Deligne, Théorie de Hodge. I, Actes du Congrès International des Mathématiciens (Nice, 1970), Tome 1, Gauthier-Villars, Paris, 1971, pp. 425–430.Google Scholar
[8] Pierre, Deligne, Théorie de Hodge. II, Inst. Hautes Études Sci. Publ. Math. (1971), no. 40, 5–57.Google Scholar
[9] Pierre, Deligne, Théorie de Hodge. III, Inst. Hautes Études Sci. Publ. Math. (1974), no. 44, 5–77.Google Scholar
[10] Jan, Denef and François, Loeser, Geometry on arc spaces of algebraic varieties, In European Congress of Mathematics, Vol. I (Barcelona, 2000), 201:327–348. Progr. Math. Basel: Birkhäuser.
[11] Jan, Denef and François, Loeser, Germs of arcs on singular algebraic varieties and motivic integration, Invent. Math. 135 (1999), 201–232, arXiv:math.AG/9803039.Google Scholar
[12] Jan, Denef and François, Loeser, Geometry on Arc Spaces, Notes of a talk available at http://www.wis.kuleuven.ac.be/algebra/NotesCambridge/.
[13] Lawrence, Ein, Robert, Lazarsfeld, and Mustaţăa, Mircea, Contact loci in arc spaces, Compos. Math. 140 (2004), no. 5, 1229–1244.Google Scholar
[14] Lawrence, Ein and Mustaţăa, Mircea, The log canonical threshold of homogeneous affine hypersurfaces, arXiv:math.AG/0105113.
[15] Lawrence, Ein and Mustaţăa, Mircea, Inversion of adjunction for local complete intersection varieties, Amer. J. Math. 126 (2004), no. 6, 1355–1365.Google Scholar
[16] Lawrence, Ein, Mustaţă, Mircea, and Yasuda, Takehiko, Jet schemes, log discrepancies and inversion of adjunction, Invent. Math. 153 (2003), no. 3, 519–535.Google Scholar
[17] David, Eisenbud, Commutative algebra, Springer-Verlag, New York, 1995.Google Scholar
[18] Marvin J., Greenberg, Schemata over local rings, Ann. of Math. (2) 73 (1961), 624–648.Google Scholar
[19] Marvin J., Greenberg, Schemata over local rings. II, Ann. of Math.(2) 78 (1963), 256–266.Google Scholar
[20] Marvin J., Greenberg, Rational points in Henselian discrete valuation rings, Inst. Hautes Études Sci. Publ. Math. (1966), no. 31, 59–64.Google Scholar
[21] Alexandre, Grothendieck, Éléments de géométrie algébrique. I. Le langage des schémas, Inst. Hautes Études Sci. Publ. Math. (1960), no. 4, 228.Google Scholar
[22] Alexandre, Grothendieck, Éléments de géométrie algébrique. IV. Étude locale des schémas et des morphismes de schémas IV, Inst. Hautes Études Sci. Publ. Math. (1967), no. 32, 361.Google Scholar
[23] Thomas C., Hales, Can p-adic integrals be computed?, Contributions to automorphic forms, geometry, and number theory, Johns Hopkins Univ. Press, Baltimore, MD, 2004, pp. 313–329.Google Scholar
[24] Thomas C., Hales, What is motivic measure?, Bull. Amer. Math. Soc. (N.S.) 42 (2005), no. 2, 119–135 (electronic).Google Scholar
[25] Robin, Hartshorne, Algebraic geometry, Springer, New York, 1973.Google Scholar
[26] Shihoko, Ishii and János, Kollár, The Nash problem on arc families of singularities, Duke Math. J. 120 (2003), no. 3, 601–620.Google Scholar
[27] Tetsushi, Ito, Stringy Hodge numbers and p-adic Hodge theory, Compos. Math. 140 (2004), no. 6, 1499–1517.Google Scholar
[28] János, Kollár, Singularities of pairs, Algebraic geometry—Santa Cruz 1995, Proc. Sympos. Pure Math., vol. 62, Amer. Math. Soc., Providence, RI, 1997, pp. 221–287.Google Scholar
[29] János, Kollár and Shigefumi, Mori, Birational geometry of algebraic varieties, Cambridge Tracts in Mathematics, vol. 134, Cambridge University Press, Cambridge, 1998, With the collaboration of C. H. Clemens and A. Corti, Translated from the 1998 Japanese original.Google Scholar
[30] Maxim, Kontsevich, Lecture at Orsay, 1995.
[31] Robert, Lazarsfeld, Positivity in algebraic geometry. II, Ergebnisse der Mathematik und ihrer Grenzgebiete. 3. Folge. A Series of Modern Surveys in Mathematics [Results in Mathematics and Related Areas. 3rd Series. A Series of Modern Surveys in Mathematics], vol. 49, Springer-Verlag, Berlin, 2004, Positivity for vector bundles, and multiplier ideals.Google Scholar
[32] Eduard, Looijenga, Motivic measures, Astérisque (2002), no. 276, 267–297, Séminaire Bourbaki, Vol. 1999/2000.Google Scholar
[33] Mustaţăa, Mircea, Jet Schemes of Locally Complete Intersection Canonical Singularities, Invent. Math. 145 (2001), 397–424, arXiv:math.AG/0008002.CrossRefGoogle Scholar
[34] Mustaţăa, Mircea, Singularities of Pairs via Jet Schemes, J. Amer. Math. Soc. 15 (2002), 599–615, arXiv:math.AG/0102201.CrossRefGoogle Scholar
[35] John F., Nash Jr., Arc structure of singularities, DukeMath.J. 81 (1995), no. 1, 31–38 (1996), A celebration of John F. Nash, Jr.Google Scholar
[36] Niko, Naumann, Algebraic independence in the Grothendieck ring of varieties, Transactions of the American Mathematical Society 359, no. 4: 1653–1683 (electronic)
[37] Bjorn, Poonen, The Grothendieck ring of varieties is not a domain, Math. Res. Lett. 9 (2002), no. 4, 493–497.Google Scholar
[38] Miles, Reid, La correspondance de McKay, Astérisque (2002), no. 276, 53–72, Séminaire Bourbaki, Vol. 1999/2000.Google Scholar
[39] Julien, Sebag, Intégration motivique sur les schémas formels, Bull. Soc. Math. France 132 (2004), no. 1, 1–54.Google Scholar
[40] Vyacheslav V., Shokurov, Letters of a bi-rationalist. IV. Geometry of log flips, Algebraic geometry, de Gruyter, Berlin, 2002, pp. 313–328.Google Scholar
[41] Shunsuke, Takagi, F-singularities of pairs and Inversion of Adjunction of arbitrary codimension, Invent. Math. 157 (2004), no. 1, 123–146.Google Scholar
[42] Willem, Veys, Arc spaces, motivic integration and stringy invariants. In: S., Izumiya et al. (eds.), Singularity Theory and its applications, pages 529–572. Volume 43 of Advanced Studies in Pure Mathematics. Mathematical Society of Japan, Tokyo, 2006.Google Scholar
[43] Takehiko, Yasuda, Dimensions of jet schemes of log singularities, Amer. J. Math. 125 (2003), no. 5, 1137–1145.Google Scholar

Save book to Kindle

To save this book to your Kindle, first ensure no-reply@cambridge.org is added to your Approved Personal Document E-mail List under your Personal Document Settings on the Manage Your Content and Devices page of your Amazon account. Then enter the ‘name’ part of your Kindle email address below. Find out more about saving to your Kindle.

Note you can select to save to either the @free.kindle.com or @kindle.com variations. ‘@free.kindle.com’ emails are free but can only be saved to your device when it is connected to wi-fi. ‘@kindle.com’ emails can be delivered even when you are not connected to wi-fi, but note that service fees apply.

Find out more about the Kindle Personal Document Service.

Available formats
×

Save book to Dropbox

To save content items to your account, please confirm that you agree to abide by our usage policies. If this is the first time you use this feature, you will be asked to authorise Cambridge Core to connect with your account. Find out more about saving content to Dropbox.

Available formats
×

Save book to Google Drive

To save content items to your account, please confirm that you agree to abide by our usage policies. If this is the first time you use this feature, you will be asked to authorise Cambridge Core to connect with your account. Find out more about saving content to Google Drive.

Available formats
×