No CrossRef data available.
Published online by Cambridge University Press: 01 July 2016
In a wide variety of multi-wave panel studies in economics and sociology, comparisons between the observed transition matrices and predictions of them based on time-homogeneous Markov chains have revealed a special kind of discrepancy: the trace of the observed matrices tends to be larger than the trace of the predicted matrices. A common explanation for this discrepancy has been via mixtures of Markov chains.
Specializing to mixtures of Markov semi-groups of the form

we exhibit classes of stochastic matrices M, probability measures µ and time intervals Δ such that
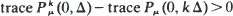 for k = 2, 3 and 4. These examples contradict the substantial literature which suggests — implicitly — that the above inequality should be reversed for general mixtures of Markov semi-groups.
for k = 2, 3 and 4. These examples contradict the substantial literature which suggests — implicitly — that the above inequality should be reversed for general mixtures of Markov semi-groups.