Article contents
Dispersive ordering results
Published online by Cambridge University Press: 01 July 2016
Abstract
A distribution F is less dispersed than a distribution G if  for all
for all 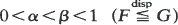 .
.
We generalize a characterization of dispersive ordering of Shaked (1982) concerning sign changes of Fc – G, where Fc is a translate of F. We then use this generalization plus total positivity to develop a simple proof of a characterization of dispersive distributions due to Lewis and Thompson (1981); a distribution H is dispersive if 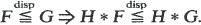
Information
- Type
- Letters to the Editor
- Information
- Copyright
- Copyright © Applied Probability Trust 1983
Footnotes
Research supported under Grant DAAG 29-82-K-0168 with the US Army Research Office.
†Postal address: Department of Statistics and Statistical Consulting Center, The Florida State University, Tallahassee, FL 32306, U.S.A.
Research supported under Contract AFOSR-82-K-0007 with the Air Force Office of Scientific Research, AFSC, USAF.
References
- 9
- Cited by

