Crossref Citations
This article has been cited by the following publications. This list is generated based on data provided by
Crossref.
Daniels, Henry E.
1990.
Stochastic Processes in Epidemic Theory.
Vol. 86,
Issue. ,
p.
129.
Lefèvre, Claude
1990.
Stochastic Processes in Epidemic Theory.
Vol. 86,
Issue. ,
p.
1.
Louchard, Guy
Kenyon, Claire
and
Schott, René
1991.
Fundamentals of Computation Theory.
Vol. 529,
Issue. ,
p.
339.
Rackwitz, R.
1992.
Reliability-Based Design of Engineered Wood Structures.
p.
47.
Phoenix, S.L.
and
Raj, R.
1992.
Overview no. 100 Scalings in fracture probabilities for a brittle matrix fiber composite.
Acta Metallurgica et Materialia,
Vol. 40,
Issue. 11,
p.
2813.
Harlow, D.G.
and
Yukich, J.E.
1993.
Empirical process methods for classical fiber bundles.
Stochastic Processes and their Applications,
Vol. 44,
Issue. 1,
p.
141.
Curtin, W.A.
1993.
The “tough” to brittle transition in brittle matrix composites.
Journal of the Mechanics and Physics of Solids,
Vol. 41,
Issue. 2,
p.
217.
Louchard, G.
1993.
TAPSOFT'93: Theory and Practice of Software Development.
Vol. 668,
Issue. ,
p.
717.
Phoenix, S.L.
1993.
Statistical issues in the fracture of brittle-matrix fibrous composites.
Composites Science and Technology,
Vol. 48,
Issue. 1-4,
p.
65.
Louchard, G.
1994.
Trie size in a dynamic list structure.
Random Structures & Algorithms,
Vol. 5,
Issue. 5,
p.
665.
Lee, Weijiang
1994.
Burst process of stretched fiber bundles.
Physical Review E,
Vol. 50,
Issue. 5,
p.
3797.
Louchard, G.
1994.
Large finite population queueing systems. The single-server model.
Stochastic Processes and their Applications,
Vol. 53,
Issue. 1,
p.
117.
Amaniampong, G.
and
Burgoyne, C.J.
1995.
Analysis of the tensile strength of parallel-lay ropes and bundles of parallel elements by probability theory.
International Journal of Solids and Structures,
Vol. 32,
Issue. 24,
p.
3573.
Gardy, Danièle
and
Louchard, Guy
1995.
Dynamic analysis of some relational databases parameters.
Theoretical Computer Science,
Vol. 144,
Issue. 1-2,
p.
125.
Ibnabdeljalil, M.
and
Phoenix, S.L.
1995.
Scalings in the statistical failure of brittle matrix composites with discontinuous fibers—I. Analysis and Monte Carlo simulations.
Acta Metallurgica et Materialia,
Vol. 43,
Issue. 8,
p.
2975.
Schäbe, Hendrik
1995.
A new approach to optimal replacement times for complex systems.
Microelectronics Reliability,
Vol. 35,
Issue. 8,
p.
1125.
Gardy, Danièle
and
Louchard, Guy
1995.
STACS 95.
Vol. 900,
Issue. ,
p.
433.
Iyengar, N.
and
Curtin, W.A.
1997.
Time-dependent failure in fiber-reinforced composites by matrix and interface shear creep.
Acta Materialia,
Vol. 45,
Issue. 8,
p.
3419.
Kloster, M.
Hansen, A.
and
Hemmer, P. C.
1997.
Burst avalanches in solvable models of fibrous materials.
Physical Review E,
Vol. 56,
Issue. 3,
p.
2615.
Sen, Pranab K.
and
Zhenwei, Zhou
1997.
Stochastic approximation and selection of a conditional extermal function in continuum.
Sequential Analysis,
Vol. 16,
Issue. 3,
p.
235.
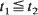 .
.
