Published online by Cambridge University Press: 29 August 2014
One of the central problems in risk theory is the calculation of the distribution function F of aggregate claims of a portfolio. Whereas formerly mainly approximation methods could be used, nowadays the increased speed of the computers allows application of iterative methods of numerical mathematics (see Bertram (1981), Küpper (1971) and Strauss (1976)). Nevertheless some of the classical approximation methods are still of some interest, especially a method developed by Esscher (1932).
The idea of this so called Esscher-approximation (see Esscher (1932), Grandell and Widaeus (1969) and Gerber (1980)) is rather simple:
In order to calculate 1 –F(x) for large x one transforms F into a distribution function 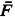 such that the mean value of
such that the mean value of 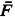 is equal to x and applies the Edgeworth expansion to the density of
is equal to x and applies the Edgeworth expansion to the density of 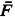 The reason for applying the transformation is the fact that the Edgeworth expansion produces good results for x near the mean value, but poor results in the tail (compare also Daniels (1954)).
The reason for applying the transformation is the fact that the Edgeworth expansion produces good results for x near the mean value, but poor results in the tail (compare also Daniels (1954)).