Crossref Citations
This article has been cited by the following publications. This list is generated based on data provided by
Crossref.
Zhao, Juan
2009.
Long time behaviour of stochastic interest rate models.
Insurance: Mathematics and Economics,
Vol. 44,
Issue. 3,
p.
459.
Dubecq, Simon
and
Gourieroux, Christian
2012.
A Term Structure Model with Level Factor Cannot Be Realistic and Arbitrage Free.
SSRN Electronic Journal,
Brody, Dorje C.
and
Hughston, L. P.
2013.
Social Discounting and the Long Rate of Interest.
SSRN Electronic Journal,
Bao, Jianhai
and
Yuan, Chenggui
2013.
Long-term behavior of stochastic interest rate models with jumps and memory.
Insurance: Mathematics and Economics,
Vol. 53,
Issue. 1,
p.
266.
De Kort, Jan
2016.
A Note on the Long Rate in Factor Models of the Term Structure.
SSRN Electronic Journal ,
Brody, Dorje C.
and
Hughston, Lane P.
2018.
SOCIAL DISCOUNTING AND THE LONG RATE OF INTEREST.
Mathematical Finance,
Vol. 28,
Issue. 1,
p.
306.
Duc, Luu Hoang
Tran, Tat Dat
and
Jost, Jürgen
2018.
Ergodicity of scalar stochastic differential equations with Hölder continuous coefficients.
Stochastic Processes and their Applications,
Vol. 128,
Issue. 10,
p.
3253.
de Kort, Jan
2018.
A note on the long rate in factor models of the term structure.
Mathematical Finance,
Vol. 28,
Issue. 2,
p.
656.
Fergusson, K.
2019.
Asymptotics of bond yields and volatilities for extended CIR models under the real-world measure.
Scandinavian Actuarial Journal,
Vol. 2019,
Issue. 10,
p.
867.
Marciniuk, Agnieszka
Zimková, Emília
Farkašovský, Vlastimil
and
Lawson, Colin W.
2020.
Valuation of Equity Release Contracts in Czech Republic, Republic of Poland and Slovak Republic.
Prague Economic Papers,
Vol. 29,
Issue. 5,
p.
505.
Roslan, Teh Raihana Nazirah
Karim, Sharmila
Ibrahim, Siti Zulaiha
Jameel, Ali Fareed
and
Yahya, Zainor Ridzuan
2021.
Stochastic pricing formulation for hybrid equity warrants.
AIMS Mathematics,
Vol. 7,
Issue. 1,
p.
398.
Baltas, I.
Szczepański, M.
Dopierala, L.
Kolodziejczyk, K.
Weber, Gerhard-Wilhelm
and
Yannacopoulos, A. N.
2021.
Modeling, Dynamics, Optimization and Bioeconomics IV.
Vol. 365,
Issue. ,
p.
31.
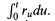 . For practice, however, this approximation turns out to be only appropriate when there are no explicit formulae and calculations are very time-consuming.
. For practice, however, this approximation turns out to be only appropriate when there are no explicit formulae and calculations are very time-consuming.

