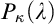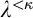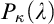Crossref Citations
This article has been cited by the following publications. This list is generated based on data provided by Crossref.
Matet, Pierre
2024.
Good points for scales (and more).
Mathematical Logic Quarterly,
Vol. 70,
Issue. 3,
p.
333.
Matet, Pierre
2025.
μ-clubs of P(λ): Paradise in heaven.
Annals of Pure and Applied Logic,
Vol. 176,
Issue. 1,
p.
103497.