Article contents
Differential Calculus and Nilpotent Real Numbers
Published online by Cambridge University Press: 15 January 2014
Extract
Do there exist real numbers d with d 2 = 0 (besides d = 0, of course)? The question is formulated provocatively, to stress a formalist view about existence: existence is consistency, or better, coherence.
Also, the provocation is meant to challenge the monopoly which the number system, invented by Dedekind et al., is claiming for itself as THE model of the geometric line. The Dedekind approach may be termed “arithmetization of geometry”.
We know that one may construct a number system out of synthetic geometry, as Euclid and followers did (completed in Hilbert's Grundlagen der Geometrie, [2, Chapter 3]): “geometrization of arithmetic”. (Picking two distinct points on the geometric line, geometric constructions in an ambient Euclidean plane provide structure of a commutative ring on the line, with the two chosen points as 0 and 1).
Starting from the geometric side, nilpotent elements are somewhat reasonable, although Euclid excluded them. The sophist Protagoras presented a picture of a circle and a tangent line; the apparent little line segment D which tangent and circle have in common, are, by Pythagoras' Theorem, precisely the points, whose abscissae d (measured along the tangent) have d 2 = 0. Protagoras wanted to use this argument for destructive reasons: to refute the science of geometry.
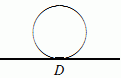
A couple of millenia later, the Danish geometer Hjelmslev revived the Protagoras picture. His aim was more positive: he wanted to describe Nature as it was. According to him (or extrapolating his position), the Real Line, the Line of Sensual Reality, had many nilpotent infinitesimals, which we can see with our naked eyes.
Information
- Type
- Research Article
- Information
- Copyright
- Copyright © Association for Symbolic Logic 2003
References
REFERENCES
- 3
- Cited by

