No CrossRef data available.
Article contents
ELLIPTIC CURVES AND 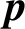 $\boldsymbol {p}$-ADIC ELLIPTIC TRANSCENDENCE
$\boldsymbol {p}$-ADIC ELLIPTIC TRANSCENDENCE
Part of:
Arithmetic algebraic geometry
Diophantine approximation, transcendental number theory
Algebraic number theory: local and $p$-adic fields
Published online by Cambridge University Press: 21 May 2021
Abstract
We prove a necessary and sufficient condition for isogenous elliptic curves based on the algebraic dependence of p-adic elliptic functions. As a consequence, we give a short proof of the p-adic analogue of Schneider’s theorem on the linear independence of p-adic elliptic logarithms of algebraic points on two nonisogenous elliptic curves defined over the field of algebraic numbers.
MSC classification
- Type
- Research Article
- Information
- Bulletin of the Australian Mathematical Society , Volume 105 , Issue 1 , February 2022 , pp. 31 - 36
- Copyright
- © 2021 Australian Mathematical Publishing Association Inc.
References
Baker, A. and Wüstholz, G.,
Logarithmic Forms and Diophantine Geometry
, New Mathematical Monographs, 9 (Cambridge University Press, Cambridge, 2007).Google Scholar
Bertrand, D., ‘Sous-groupes à un paramètre
 $p$
-adique de variétés de groupe’,
Invent. Math.
40(2) (1977), 171–193.CrossRefGoogle Scholar
$p$
-adique de variétés de groupe’,
Invent. Math.
40(2) (1977), 171–193.CrossRefGoogle Scholar
Bourbaki, N., Elements of Mathematics. Lie groups and Lie algebras. Part I: Chapters 1–3 (Hermann, Paris, 1975).Google Scholar
Fuchs, C. and Pham, D. H., ‘The
 $p$
-adic analytic subgroup theorem revisited’,
$p$
-adic analytic subgroup theorem revisited’,
 $p$
-Adic Numbers Ultrametric Anal. Appl. 7 (2015), 143–156.CrossRefGoogle Scholar
$p$
-Adic Numbers Ultrametric Anal. Appl. 7 (2015), 143–156.CrossRefGoogle Scholar
Fuchs, C. and Pham, D. H., ‘Some applications of the
 $p$
-adic analytic subgroup theorem’,
Glas. Mat.
51 (2016), 335–343.CrossRefGoogle Scholar
$p$
-adic analytic subgroup theorem’,
Glas. Mat.
51 (2016), 335–343.CrossRefGoogle Scholar
Lutz, E., ‘Sur l’équation
 ${Y}^2=A{X}^3-\mathrm{AX}-B$
dans les corps
${Y}^2=A{X}^3-\mathrm{AX}-B$
dans les corps
 $p$
-adiques’,
J. reine angew. Math.
177 (1937), 238–247.CrossRefGoogle Scholar
$p$
-adiques’,
J. reine angew. Math.
177 (1937), 238–247.CrossRefGoogle Scholar
Schneider, T., ‘Arithmetische Untersuchungen elliptischer Integrale’,
Math. Ann.
113 (1936), 1–13.CrossRefGoogle Scholar
Schneider, T., Einführung in die Transzendenten Zahlen (Springer-Verlag, Berlin, 1957).CrossRefGoogle Scholar
Weil, A., ‘Sur les fonctions elliptiques
 $p$
-adiques’,
Note aux C. R. Acad. Sci. Paris
203 (1936), 22–24.Google Scholar
$p$
-adiques’,
Note aux C. R. Acad. Sci. Paris
203 (1936), 22–24.Google Scholar
Wüstholz, G., ‘Algebraische Punkte auf analytischen Untergruppen algebraischer Gruppen’,
Ann. of Math.
129 (1989), 501–517.CrossRefGoogle Scholar
Zarhin, Y. G., ‘
 $p$
-adic abelian integrals and commutative Lie groups’,
J. Math. Sci.
81(3) (1996), 2744–2750.CrossRefGoogle Scholar
$p$
-adic abelian integrals and commutative Lie groups’,
J. Math. Sci.
81(3) (1996), 2744–2750.CrossRefGoogle Scholar



