Hostname: page-component-78c5997874-94fs2 Total loading time: 0 Render date: 2024-11-10T23:27:46.795Z Has data issue: false hasContentIssue false
  • English
  • Français

FIXED POINTS OF POLYNOMIALS OVER DIVISION RINGS

Part of: Division rings and semisimple Artin rings Rings and algebras arising under various constructions Smooth dynamical systems: general theory Arithmetic and non-Archimedean dynamical systems

Published online by Cambridge University Press:  01 March 2021

ADAM CHAPMAN Open the ORCID record for ADAM CHAPMAN [Opens in a new window]  and
SOLOMON VISHKAUTSAN Open the ORCID record for SOLOMON VISHKAUTSAN [Opens in a new window]
ADAM CHAPMAN
Affiliation:
School of Computer Science, Academic College of Tel-Aviv-Yaffo, Rabenu Yeruham St., PO Box 8401, Yaffo6818211, Israel e-mail: adam1chapman@yahoo.com
SOLOMON VISHKAUTSAN*
Affiliation:
Department of Computer Science, Tel-Hai Academic College, Upper Galilee, Qiryat Shemona1220800, Israel
*
e-mail: wishcow@gmail.com
Get access Rights & Permissions [Opens in a new window]

Abstract

We study the discrete dynamics of standard (or left) polynomials $f(x)$ over division rings D. We define their fixed points to be the points 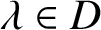$\lambda \in D$ for which $f^{\circ n}(\lambda )=\lambda $ for any 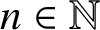$n \in \mathbb {N}$ , where 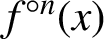$f^{\circ n}(x)$ is defined recursively by 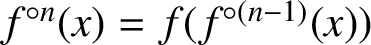$f^{\circ n}(x)=f(f^{\circ (n-1)}(x))$ and 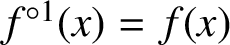$f^{\circ 1}(x)=f(x)$ . Periodic points are similarly defined. We prove that 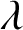$\lambda $ is a fixed point of 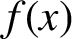$f(x)$ if and only if $f(\lambda )=\lambda $ , which enables the use of known results from the theory of polynomial equations, to conclude that any polynomial of degree 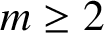$m \geq 2$ has at most m conjugacy classes of fixed points. We also show that in general, periodic points do not behave as in the commutative case. We provide a sufficient condition for periodic points to behave as expected.

Keywords

division algebradivision ringfixed pointnoncommutative dynamical systemperiodic pointpolynomial ringstandard polynomial

MSC classification

Primary: 16S36: Ordinary and skew polynomial rings and semigroup rings
Secondary: 16K20: Finite-dimensional 37C25: Fixed points, periodic points, fixed-point index theory 37P35: Arithmetic properties of periodic points
Type
Research Article
Information
Bulletin of the Australian Mathematical Society , Volume 104 , Issue 2 , October 2021 , pp. 256 - 262
Check for updates
Copyright
© 2021 Australian Mathematical Publishing Association Inc.

Access options

Get access to the full version of this content by using one of the access options below. (Log in options will check for institutional or personal access. Content may require purchase if you do not have access.)

Footnotes

The first author acknowledges the receipt of the Chateaubriand Fellowship (969845L) offered by the French Embassy in Israel.

References

Berhuy, G. and Oggier, F., An Introduction to Central Simple Algebras and their Applications to Wireless Communication, Mathematical Surveys and Monographs, 191 (American Mathematical Society, Providence, RI, 2013).CrossRefGoogle Scholar
Chapman, A., ‘Polynomial equations over octonion algebras’, J. Algebra Appl. 19(6) (2020), 2050102.CrossRefGoogle Scholar
Chapman, A. and Machen, C., ‘Standard polynomial equations over division algebras’, Adv. Appl. Clifford Algebr. 27(2) (2017), 1065–1072.CrossRefGoogle Scholar
Gordon, B. and Motzkin, T. S., ‘On the zeros of polynomials over division rings’, Trans. Amer. Math. Soc. 116 (1965), 218–226.CrossRefGoogle Scholar
Janovská, D. and Opfer, G., ‘A note on the computation of all zeros of simple quaternionic polynomials’, SIAM J. Numer. Anal. 48(1) (2010), 244–256.CrossRefGoogle Scholar
Knus, M.-A., Merkurjev, A., Rost, M. and Tignol, J.-P., The Book of Involutions, American Mathematical Society Colloquium Publications, 44 (American Mathematical Society, Providence, RI, 1998).CrossRefGoogle Scholar
Lam, T. Y., Leroy, A. and Ozturk, A., ‘Wedderburn polynomials over division rings. II’, in: Noncommutative Rings, Group Rings, Diagram Algebras and Their Applications, Contemporary Mathematics, 456 (American Mathematical Society, Providence, RI, 2008), 73–98.CrossRefGoogle Scholar
Loos, O., Petersson, H. P. and Racine, M. L., ‘Inner derivations of alternative algebras over commutative rings’, Algebra Number Theory 2(8) (2008), 927–968.CrossRefGoogle Scholar
Silverman, J. H., The Arithmetic of Dynamical Systems, Graduate Texts in Mathematics, 241 (Springer, New York, 2007).CrossRefGoogle Scholar
Zhevlakov, K. A., Slin’ko, A. M., Shestakov, I. P. and Shirshov, A. I., Rings that Are Nearly Associative, Pure and Applied Mathematics, 104 (Academic Press, Inc. [Harcourt Brace Jovanovich, Publishers], New York, 1982).Google Scholar