Published online by Cambridge University Press: 17 April 2009
Consider a bounded linear operator T between Banach spaces ℬ, ℬ′ which can be decomposed into direct sums ℬ = ℬ1 ⌖ ℬ2, ℬ′ = ℬ1′ ⌖ ℬ2′. Such linear operator can be represented by a 2 × 2 operator matrix of the form
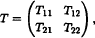 where Tij ∈ ℒ(ℬj, ℒi′) i, j = 1, 2. (By ℒ(ℬj, ℒi′) we denote the space of bounded linear operators acting from ℬj to ℬi′ (i, j = 1, 2).) The map GT from L (B1, B2) into the set of closed affine subspaces of ℒ(ℬ1′ ℬ2′), defined by
where Tij ∈ ℒ(ℬj, ℒi′) i, j = 1, 2. (By ℒ(ℬj, ℒi′) we denote the space of bounded linear operators acting from ℬj to ℬi′ (i, j = 1, 2).) The map GT from L (B1, B2) into the set of closed affine subspaces of ℒ(ℬ1′ ℬ2′), defined by
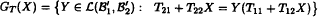 is called a linear fractional relation associated with T.
is called a linear fractional relation associated with T.
Such relations can be considered as a generalisation of linear fractional transformations which were studied by many authors and found many applications. Many traditional and recently discovered areas of application of linear fractional transformations would benefit from a better understanding of the behaviour of linear fractional relations. The present paper is devoted to analogues of the Liouville theorem “a bounded entire function is constant” for linear fractional relations.