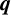Crossref Citations
This article has been cited by the following publications. This list is generated based on data provided by Crossref.
Wei, Chuanan
and
Wang, Qin
2025.
A q-Analog of Deines, Fuselier, Long, Swisher, and Tu’s Supercongruence.
Mediterranean Journal of Mathematics,
Vol. 22,
Issue. 5,
Hu, Yu
and
Wang, Xiaoxia
2025.
Proof of a conjecture by Wei.
Bulletin of the Malaysian Mathematical Sciences Society,
Vol. 48,
Issue. 4,
Guo, Victor J. W.
2025.
Proof of a supercongruence modulo p2r$p^{2r}$.
Bulletin of the London Mathematical Society,