Hostname: page-component-78c5997874-dh8gc Total loading time: 0 Render date: 2024-11-13T02:44:06.287Z Has data issue: false hasContentIssue false
  • English
  • Français

BOUNDS ON MINORS OF BINARY MATRICES

Part of: Designs and configurations Special matrices

Published online by Cambridge University Press:  23 December 2012

RICHARD P. BRENT  and
JUDY-ANNE H. OSBORN
RICHARD P. BRENT*
Affiliation:
Australian National University, Canberra, ACT 0200, Australia (email: minors@rpbrent.com)
JUDY-ANNE H. OSBORN
Affiliation:
The University of Newcastle, Callaghan, NSW 2308, Australia (email: Judy-anne.Osborn@newcastle.edu.au)
*
✉For correspondence; e-mail: minors@rpbrent.com
Rights & Permissions [Opens in a new window]

Abstract

Core share and HTML view are not available for this content. However, as you have access to this content, a full PDF is available via the ‘Save PDF’ action button.

We prove an upper bound on sums of squares of minors of 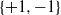$\{+1, -1\}$-matrices. The bound is sharp for Hadamard matrices, a result due to de Launey and Levin [‘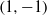$(1,-1)$-matrices with near-extremal properties’, SIAM J. Discrete Math.23(2009), 1422–1440], but our proof is simpler. We give several corollaries relevant to minors of Hadamard matrices.

Keywords

Hadamard matricesbinary matrices{+1−1}-matricesminorsCauchy–Binet formulamean square

MSC classification

Secondary: 05B20: Matrices (incidence, Hadamard, etc.) 15B34: Boolean and Hadamard matrices
Type
Research Article
Information
Bulletin of the Australian Mathematical Society , Volume 88 , Issue 2 , October 2013 , pp. 280 - 285
Copyright
Copyright © 2012 Australian Mathematical Publishing Association Inc. 

References

[1]Brualdi, R. A. & Schneider, H., ‘Determinantal identities: Gauss, Schur, Cauchy, Sylvester, Kronecker, Jacobi, Binet, Laplace, Muir, and Cayley’, Linear Algebra Appl. 52/53 (1983), 769–791.CrossRefGoogle Scholar
[2]de Launey, W. & Levin, D. A., ‘$(1,-1)$-matrices with near-extremal properties’, SIAM J. Discrete Math. 23 (2009), 1422–1440.CrossRefGoogle Scholar
[3]Gantmacher, F. R., The Theory of Matrices, Vol. 1 (AMS Chelsea Publishing, Providence, RI, 2000).Google Scholar
[4]Hadamard, J., ‘Résolution d’une question relative aux déterminants’, Bull. Sci. Math. 17 (1893), 240–246.Google Scholar
[5]Jacobi, C. G. J., ‘De formatione et proprietatibus determinantium’, Crelle’s J. 22 (1841), 285–318; also Gesammelte Werke, Bd. 3 (Chelsea Publishing Company, New York, 1969).Google Scholar
[6]Kahn, J., Komlós, J. & Szemerédi, E., ‘On the probability that a random $\pm 1$-matrix is singular’, J. Amer. Math. Soc. 8 (1995), 223–240.Google Scholar
[7]Koukouvinos, C., Lappas, E., Mitrouli, M. & Seberry, J., ‘An algorithm to find formulae and values of minors for Hadamard matrices: II’, Linear Algebra Appl. 371 (2003), 111–124.CrossRefGoogle Scholar
[8]Koukouvinos, C., Mitrouli, M. & Seberry, J., ‘An algorithm to find formulae and values of minors for Hadamard matrices’, Linear Algebra Appl. 330 (2001), 129–147.CrossRefGoogle Scholar
[9]Little, C. H. C. & Thuente, D. J., ‘The Hadamard conjecture and circuits of length four in a complete bipartite graph’, J. Aust. Math. Soc. (Ser. A) 31 (1981), 252–256.CrossRefGoogle Scholar
[10]Muir, T., A Treatise on the Theory of Determinants (Dover, New York, 1960).Google Scholar
[11]OEIS Foundation Inc., ‘The on-line encyclopedia of integer sequences’, 2012,http://oeis.org/A057982. Also A046747.Google Scholar
[12]Sharpe, F. R., ‘The maximum value of a determinant’, Bull. Amer. Math. Soc. 14 (1907), 121–123.CrossRefGoogle Scholar
[13]Szöllősi, F., ‘Exotic complex Hadamard matrices and their equivalence’, Cryptogr. Commun. 2 (2010), 187–198.CrossRefGoogle Scholar
[14]Tao, T. & Vu, V., ‘On random $\pm 1$ matrices: singularity and determinant’, Random Structures Algorithms 28 (2006), 1–23.CrossRefGoogle Scholar
[15]Turán, P., ‘On extremal problems concerning determinants’, Math. Naturwiss. Anz. Ungar. Akad. Wiss. 59 (1940), 95–105.Google Scholar