Hostname: page-component-cd9895bd7-gvvz8 Total loading time: 0 Render date: 2024-12-28T01:26:48.591Z Has data issue: false hasContentIssue false
  • English
  • Français

COMPARING THE GENERALISED ROUNDNESS OF METRIC SPACES

Part of: Graph theory Normed linear spaces and Banach spaces; Banach lattices

Published online by Cambridge University Press:  02 November 2016

LUKIEL LEVY-MOORE ,
MARGARET NICHOLS  and
ANTHONY WESTON
LUKIEL LEVY-MOORE
Affiliation:
Department of Economics, New York University, New York, NY 10012, USA email LukielLevyMoore@nyu.edu
MARGARET NICHOLS
Affiliation:
Department of Mathematics, University of Chicago, Chicago, IL 60637, USA email mnichols@math.uchicago.edu
ANTHONY WESTON*
Affiliation:
Department of Mathematics and Statistics, Canisius College, Buffalo, NY 14208, USA Department of Decision Sciences, University of South Africa, Unisa 0003, South Africa email westona@canisius.edu, westoar@unisa.ac.za
*
✉westona@canisius.edu
Rights & Permissions [Opens in a new window]

Abstract

Core share and HTML view are not available for this content. However, as you have access to this content, a full PDF is available via the ‘Save PDF’ action button.

Motivated by the local theory of Banach spaces, we introduce a notion of finite representability for metric spaces. This allows us to develop a new technique for comparing the generalised roundness of metric spaces. We illustrate this technique by applying it to Banach spaces and metric trees. In the realm of Banach spaces we obtain results such as the following: (1) if 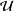${\mathcal{U}}$ is any ultrafilter and 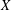$X$ is any Banach space, then the second dual 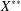$X^{\ast \ast }$ and the ultrapower 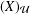$(X)_{{\mathcal{U}}}$ have the same generalised roundness as 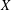$X$, and (2) no Banach space of positive generalised roundness is uniformly homeomorphic to 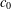$c_{0}$ or 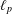$\ell _{p}$, 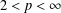$2<p<\infty$. For metric trees, we give the first examples of metric trees of generalised roundness one that have finite diameter. In addition, we show that metric trees of generalised roundness one possess special Euclidean embedding properties that distinguish them from all other metric trees.

Keywords

generalised roundnessnegative typeuniform homeomorphismscale isomorphism

MSC classification

Primary: 46B07: Local theory of Banach spaces
Secondary: 05C05: Trees 05C12: Distance in graphs 46B80: Nonlinear classification of Banach spaces; nonlinear quotients
Type
Research Article
Information
Bulletin of the Australian Mathematical Society , Volume 95 , Issue 2 , April 2017 , pp. 299 - 314
Copyright
© 2016 Australian Mathematical Publishing Association Inc. 

Footnotes

The research in this paper was initiated at the 2011 Cornell University Summer Mathematics Institute (NSF grant DMS-0739338) and completed at the University of South Africa (Unisa). The second named author was partially supported by the National Science Foundation Graduate Research Fellowship Program (NSF grant DGE-1144082).

References

Bird, A., Jameson, G. and Laustsen, N. J., ‘The Giesy–James theorem for general index p, with an application to operator ideals on the pth James space’, J. Operator Theory 70 (2013), 291–307.CrossRefGoogle Scholar
Caffarelli, E., Doust, I. and Weston, A., ‘Metric trees of generalized roundness one’, Aequationes Math. 83 (2012), 239–256.CrossRefGoogle Scholar
Cayley, A., ‘On a theorem in the geometry of position’, Cambridge Math. J. II (1841), 267–271. Also in The Collected Mathematical Papers of Arthur Cayley, Vol. I (Cambridge University Press, Cambridge, 1889), 1–4.Google Scholar
Dahma, A. M. and Lennard, C. J., ‘Generalized roundness of the Schatten class, C p ’, J. Math. Anal. Appl. 412 (2014), 676–684.CrossRefGoogle Scholar
Diestel, J., Jarchow, H. and Tonge, A. M., Absolutely Summing Operators, Cambridge Studies in Advanced Mathematics, 43 (Cambridge University Press, Cambridge, 1995).CrossRefGoogle Scholar
Dineen, S., ‘A Dvoretzky theorem for polynomials’, Proc. Amer. Math. Soc. 123 (1995), 2817–2821.Google Scholar
Dranishnikov, A. N., Gong, G., Lafforgue, V. and Yu, G., ‘Uniform embeddings into Hilbert space and a question of Gromov’, Canad. Math. Bull. 45 (2002), 60–70.CrossRefGoogle Scholar
Enflo, P., ‘On a problem of Smirnov’, Ark. Mat. 8 (1969), 107–109.CrossRefGoogle Scholar
Enflo, P., ‘Uniform structures and square roots in topological spaces II’, Israel J. Math. 8 (1970), 253–272.CrossRefGoogle Scholar
Faver, T., Kochalski, K., Murugan, M., Verheggen, H., Wesson, E. and Weston, A., ‘Roundness properties of ultrametric spaces’, Glasg. Math. J. 56 (2014), 519–535.CrossRefGoogle Scholar
Giesy, D. P. and James, R. C., ‘Uniformly non-ℓ 1 and B-convex Banach spaces’, Studia Math. 48 (1973), 61–69.CrossRefGoogle Scholar
Godefroy, G., Kalton, N. J. and Lancien, G., ‘Szlenk indices and uniform homeomorphisms’, Trans. Amer. Math. Soc. 353 (2001), 3895–3918.CrossRefGoogle Scholar
Hájek, P. and Johanis, M., Smooth Analysis in Banach Spaces, De Gruyter Series in Nonlinear Analysis and Applications, 19 (De Gruyter, Berlin, 2014), 1–497.CrossRefGoogle Scholar
Hjorth, P., Lisoněk, P., Markvorsen, S. and Thomassen, C., ‘Finite metric spaces of strictly negative type’, Linear Algebra Appl. 270 (1998), 255–273.CrossRefGoogle Scholar
James, R. C., ‘Uniformly nonsquare Banach spaces’, Ann. of Math. (2) 80 (1964), 542–550.CrossRefGoogle Scholar
James, R. C., ‘Some self-dual properties of normed linear spaces’, in: Sympos. Infinite Dimensional Topology, Annals of Mathematics Studies, 69 (Princeton University Press, Princeton, NJ, 1972).Google Scholar
James, R. C., ‘Super-reflexive Banach spaces’, Canad. J. Math. 24 (1972), 896–904.CrossRefGoogle Scholar
Johnson, W. B., Lindenstrauss, J. and Schechtman, G., ‘Banach spaces determined by their linear structure’, Geom. Funct. Anal. 6 (1996), 430–470.CrossRefGoogle Scholar
Kelleher, C., Miller, D., Osborn, T. and Weston, A., ‘Strongly non-embeddable metric spaces’, Topology Appl. 159 (2011), 749–755.CrossRefGoogle Scholar
Kelleher, C., Miller, D., Osborn, T. and Weston, A., ‘Polygonal equalities and virtual degeneracy in L p -spaces’, J. Math. Anal. Appl. 415 (2014), 247–268.CrossRefGoogle Scholar
Kelly, J. B., ‘Hypermetric spaces’, in: Proc. Conf. Geometry of Metric and Linear Spaces, Lecture Notes in Mathematics, 490 (Springer, Berlin, 1975), 17–31.CrossRefGoogle Scholar
Krivine, J. L., ‘Sous-espaces de dimension finie des espaces de Banach réticulé’, Ann. of Math. (2) 104 (1976), 1–29.CrossRefGoogle Scholar
Lafont, J.-F. and Prassidis, S., ‘Roundness properties of groups’, Geom. Dedicata 117 (2006), 137–160.CrossRefGoogle Scholar
Lemberg, H., ‘Nouvelle démonstration d’un théorème de J. L. Krivine sur la finie représentation de ℓ p dans un espace de Banach’, Israel J. Math. 39 (1981), 341–348.CrossRefGoogle Scholar
Lennard, C. J., Tonge, A. M. and Weston, A., ‘Generalized roundness and negative type’, Michigan Math. J. 44 (1997), 37–45.CrossRefGoogle Scholar
Lennard, C. J., Tonge, A. M. and Weston, A., ‘Roundness and metric type’, J. Math. Anal. Appl. 252 (2000), 980–988.CrossRefGoogle Scholar
Li, H. and Weston, A., ‘Strict p-negative type of a metric space’, Positivity 14 (2010), 529–545.CrossRefGoogle Scholar
Lindenstrauss, J. and Rosenthal, H., ‘The L p spaces’, Israel J. Math. 7 (1969), 325–349.CrossRefGoogle Scholar
Maurey, B. and Pisier, G., ‘Séries de variables aléatoires vectorielles indépendantes et propriétés géométriques des espaces de Banach’, Studia Math. 58 (1976), 45–90.CrossRefGoogle Scholar
Prassidis, E. and Weston, A., ‘Manifestations of non linear roundness in analysis, discrete geometry and topology’, in: Limits of Graphs in Group Theory and Computer Science, Research Proceedings of the École Polytechnique Fédérale de Lausanne (eds. Arzhantseva, G. and Valette, A.) (CRC Press, Boca Raton, FL, 2009), 141–170.Google Scholar
Ribe, M., ‘On uniformly homeomorphic normed spaces’, Ark. Mat. 14 (1976), 237–244.CrossRefGoogle Scholar
Rosenthal, H. P., ‘On a theorem of J. L. Krivine concerning block finite representability of ℓ p in general Banach spaces’, J. Funct. Anal. 28 (1978), 197–225.CrossRefGoogle Scholar
Sánchez, S., ‘On the supremal p-negative type of a finite metric space’, J. Math. Anal. Appl. 389 (2012), 98–107.CrossRefGoogle Scholar
Schoenberg, I. J., ‘On certain metric spaces arising from Euclidean spaces by a change of metric and their imbedding in Hilbert space’, Ann. of Math. (2) 38 (1937), 787–793.CrossRefGoogle Scholar
Stern, J., ‘Some applications of model theory in Banach space theory’, Ann. Math. Logic 9 (1976), 49–122.CrossRefGoogle Scholar
Weston, A., ‘On the generalized roundness of finite metric spaces’, J. Math. Anal. Appl. 192 (1995), 323–334.CrossRefGoogle Scholar