Hostname: page-component-cd9895bd7-hc48f Total loading time: 0 Render date: 2024-12-27T07:08:18.716Z Has data issue: false hasContentIssue false
  • English
  • Français

DICHOTOMY PROPERTY FOR MAXIMAL OPERATORS IN A NONDOUBLING SETTING

Part of: Harmonic analysis in several variables Metric geometry

Published online by Cambridge University Press:  26 December 2018

DARIUSZ KOSZ Open the ORCID record for DARIUSZ KOSZ [Opens in a new window]
DARIUSZ KOSZ*
Affiliation:
Faculty of Pure and Applied Mathematics, Wrocław University of Science and Technology, Wyb. Wyspiańskiego 27, 50-370 Wrocław, Poland email Dariusz.Kosz@pwr.edu.pl
Rights & Permissions [Opens in a new window]

Abstract

Core share and HTML view are not available for this content. However, as you have access to this content, a full PDF is available via the ‘Save PDF’ action button.

We investigate a dichotomy property for Hardy–Littlewood maximal operators, noncentred 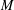$M$ and centred 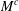$M^{c}$, that was noticed by Bennett et al. [‘Weak-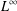$L^{\infty }$ and BMO’, Ann. of Math. (2) 113 (1981), 601–611]. We illustrate the full spectrum of possible cases related to the occurrence or not of this property for $M$ and $M^{c}$ in the context of nondoubling metric measure spaces $(X,\unicode[STIX]{x1D70C},\unicode[STIX]{x1D707})$. In addition, if 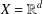$X=\mathbb{R}^{d}$, 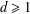$d\geq 1$, and 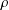$\unicode[STIX]{x1D70C}$ is the metric induced by an arbitrary norm on $\mathbb{R}^{d}$, then we give the exact characterisation (in terms of $\unicode[STIX]{x1D707}$) of situations in which $M^{c}$ possesses the dichotomy property provided that 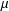$\unicode[STIX]{x1D707}$ satisfies some very mild assumptions.

Keywords

maximal operatordichotomy propertymetric measure spacenondoubling measure

MSC classification

Primary: 42B25: Maximal functions, Littlewood-Paley theory
Secondary: 51F99: None of the above, but in this section
Type
Research Article
Information
Bulletin of the Australian Mathematical Society , Volume 99 , Issue 3 , June 2019 , pp. 454 - 466
Copyright
© 2018 Australian Mathematical Publishing Association Inc. 

Footnotes

The author is supported by the National Science Centre of Poland, project no. 2016/21/N/ST1/01496.

References

Aalto, D. and Kinnunen, J., ‘The discrete maximal operator in metric spaces’, J. Anal. Math. 111 (2010), 369–390.Google Scholar
Bennett, C., DeVore, R. A. and Sharpley, R., ‘Weak-L ∞ and BMO’, Ann. of Math. (2) 113 (1981), 601–611.Google Scholar
Federer, H., Geometric Measure Theory (Springer, New York, 1969).Google Scholar
Fiorenza, A. and Krbec, M., ‘On the domain and range of the maximal operator’, Nagoya Math. J. 158 (2000), 43–61.Google Scholar
Folland, G. B., Real Analysis: Modern Techniques and Their Applications (Wiley, New York, 1999).Google Scholar
Hytönen, T., ‘A framework for non-homogeneous analysis on metric spaces, and the RBMO space of Tolsa’, Publ. Mat. 54(2) (2010), 485–504.Google Scholar
Lin, C.-C., Stempak, K. and Wang, Y.-S., ‘Local maximal operators on measure metric spaces’, Publ. Mat. 57(1) (2013), 239–264.Google Scholar