No CrossRef data available.
Article contents
Dirichlet series with positive real part
Published online by Cambridge University Press: 17 April 2009
Abstract
We consider the sequence Λ = {0 < λ2 < λ2 < …}, for which λn → +∞. We denote by PD(Λ) the class of Dirichlet's series having the form F(s) = 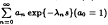 defined in the half plan Re s > 0 converging absolutely and Re F ≥ 0. If N0 = {0, 1,2, …} then the class PD(N0) coincides with the Caratheodory's class P. In this paper some classical results holding for the class P are generalised in any class PD(Λ). In special cases for the sequence Λ extreme problems are examined in the class PD(Λ).
defined in the half plan Re s > 0 converging absolutely and Re F ≥ 0. If N0 = {0, 1,2, …} then the class PD(N0) coincides with the Caratheodory's class P. In this paper some classical results holding for the class P are generalised in any class PD(Λ). In special cases for the sequence Λ extreme problems are examined in the class PD(Λ).
- Type
- Research Article
- Information
- Copyright
- Copyright © Australian Mathematical Society 1992


