Article contents
Existence and multiplicity results for quasilinear elliptic equations
Published online by Cambridge University Press: 17 April 2009
Extract
The goal of this paper is to study the multiplicity of positive solutions of a class of quasilinear elliptic equations. Based on the mountain pass theorems and sub-and supersolutions argument for p-Laplacian operators, under suitable conditions on nonlinearity f(x, s), we show the follwing problem:
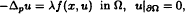 ,
where Ω is a bounded open subset of RN, N ≥ 2, with smooth boundary, λ is a positive parameter and ∆p is the p-Laplacian operator with p > 1, possesses at least two positive solutions for large λ.
,
where Ω is a bounded open subset of RN, N ≥ 2, with smooth boundary, λ is a positive parameter and ∆p is the p-Laplacian operator with p > 1, possesses at least two positive solutions for large λ.
- Type
- Research Article
- Information
- Copyright
- Copyright © Australian Mathematical Society 2005
References
- 1
- Cited by


