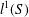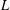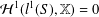Crossref Citations
This article has been cited by the following publications. This list is generated based on data provided by Crossref.
Minapoor, Ahmad
and
Zivari-Kazempour, Abbas
2023.
Ideal Connes-Amenability of Certain Dual Banach Algebras.
Complex Analysis and Operator Theory,
Vol. 17,
Issue. 2,