No CrossRef data available.
Article contents
THE FLOOR OF THE ARITHMETIC MEAN OF THE CUBE ROOTS OF THE FIRST  $n$ INTEGERS
$n$ INTEGERS
Published online by Cambridge University Press: 08 January 2020
Abstract
Zacharias [‘Proof of a conjecture of Merca on an average of square roots’, College Math. J.49 (2018), 342–345] proved Merca’s conjecture that the arithmetic means 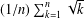 $(1/n)\sum _{k=1}^{n}\sqrt{k}$ of the square roots of the first
$(1/n)\sum _{k=1}^{n}\sqrt{k}$ of the square roots of the first  $n$ integers have the same floor values as a simple approximating sequence. We prove a similar result for the arithmetic means
$n$ integers have the same floor values as a simple approximating sequence. We prove a similar result for the arithmetic means 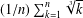 $(1/n)\sum _{k=1}^{n}\sqrt[3]{k}$ of the cube roots of the first
$(1/n)\sum _{k=1}^{n}\sqrt[3]{k}$ of the cube roots of the first  $n$ integers.
$n$ integers.
Keywords
MSC classification
- Type
- Research Article
- Information
- Bulletin of the Australian Mathematical Society , Volume 102 , Issue 2 , October 2020 , pp. 261 - 267
- Copyright
- © 2020 Australian Mathematical Publishing Association Inc.
Footnotes
The authors were supported by the Faculty of Science, Burapha University, Thailand.



