Article contents
A GROUP SUM INEQUALITY AND ITS APPLICATION TO POWER GRAPHS
Published online by Cambridge University Press: 13 June 2014
Abstract
Let 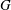 $\def \xmlpi #1{}\def \mathsfbi #1{\boldsymbol {\mathsf {#1}}}\let \le =\leqslant \let \leq =\leqslant \let \ge =\geqslant \let \geq =\geqslant \def \Pr {\mathit {Pr}}\def \Fr {\mathit {Fr}}\def \Rey {\mathit {Re}}G$ be a finite group of order
$\def \xmlpi #1{}\def \mathsfbi #1{\boldsymbol {\mathsf {#1}}}\let \le =\leqslant \let \leq =\leqslant \let \ge =\geqslant \let \geq =\geqslant \def \Pr {\mathit {Pr}}\def \Fr {\mathit {Fr}}\def \Rey {\mathit {Re}}G$ be a finite group of order  $n$, and let
$n$, and let 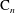 $\text {C}_n$ be the cyclic group of order
$\text {C}_n$ be the cyclic group of order  $n$. For
$n$. For 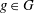 $g\in G$, let
$g\in G$, let 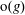 ${\mathrm{o}}(g)$ denote the order of
${\mathrm{o}}(g)$ denote the order of  $g$. Let
$g$. Let 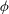 $\phi $ denote the Euler totient function. We show that
$\phi $ denote the Euler totient function. We show that 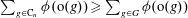 $\sum _{g \in \text {C}_n} \phi ({\mathrm{o}}(g))\geq \sum _{g \in G} \phi ({\mathrm{o}}(g))$, with equality if and only if
$\sum _{g \in \text {C}_n} \phi ({\mathrm{o}}(g))\geq \sum _{g \in G} \phi ({\mathrm{o}}(g))$, with equality if and only if 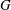 $G$ is isomorphic to
$G$ is isomorphic to 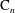 $\text {C}_n$. As an application, we show that among all finite groups of a given order, the cyclic group of that order has the maximum number of bidirectional edges in its directed power graph.
$\text {C}_n$. As an application, we show that among all finite groups of a given order, the cyclic group of that order has the maximum number of bidirectional edges in its directed power graph.
MSC classification
Information
- Type
- Research Article
- Information
- Bulletin of the Australian Mathematical Society , Volume 90 , Issue 3 , December 2014 , pp. 418 - 426
- Copyright
- Copyright © 2014 Australian Mathematical Publishing Association Inc.
References
- 3
- Cited by

