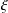Crossref Citations
This article has been cited by the following publications. This list is generated based on data provided by Crossref.
Pawelec, Łukasz
and
Urbański, Mariusz
2020.
Long hitting times for expanding systems.
Nonlinearity,
Vol. 33,
Issue. 3,
p.
942.
Kleinbock, Dmitry
and
Zheng, Jiajie
2023.
Dynamical Borel–Cantelli lemma for recurrence under Lipschitz twists.
Nonlinearity,
Vol. 36,
Issue. 2,
p.
1434.
Pawelec, Łukasz
2024.
Estimating the Hausdorff measure using recurrence.
Proceedings of the Edinburgh Mathematical Society,
Vol. 67,
Issue. 1,
p.
188.
RODRIGUEZ SPONHEIMER, ALEJANDRO
2025.
A recurrence-type strong Borel–Cantelli lemma for Axiom A diffeomorphisms.
Ergodic Theory and Dynamical Systems,
Vol. 45,
Issue. 3,
p.
936.