Hostname: page-component-cd9895bd7-fscjk Total loading time: 0 Render date: 2024-12-27T06:06:18.830Z Has data issue: false hasContentIssue false
  • English
  • Français

KRASNOSELSKI–MANN ITERATION FOR HIERARCHICAL FIXED POINTS AND EQUILIBRIUM PROBLEM

Part of: Nonlinear operators and their properties

Published online by Cambridge University Press:  26 February 2009

GIUSEPPE MARINO ,
VITTORIO COLAO ,
LUIGI MUGLIA  and
YONGHONG YAO
GIUSEPPE MARINO*
Affiliation:
Dipartimento di Matematica, Università della Calabria, 87036, Arcavacata di Rende (CS), Italy (email: gmarino@unical.it)
VITTORIO COLAO
Affiliation:
Dipartimento di Matematica, Università della Calabria, 87036, Arcavacata di Rende (CS), Italy (email: colao@mat.unical.it)
LUIGI MUGLIA
Affiliation:
Dipartimento di Matematica, Università della Calabria, 87036, Arcavacata di Rende (CS), Italy (email: muglia@mat.unical.it)
YONGHONG YAO
Affiliation:
Department of Mathematics, Tianjin Polytechnic University, Tianjin 300160, People’s Republic of China (email: yaoyonghong@yahoo.cn)
*
✉For correspondence; e-mail: gmarino@unical.it
Rights & Permissions [Opens in a new window]

Abstract

Core share and HTML view are not available for this content. However, as you have access to this content, a full PDF is available via the ‘Save PDF’ action button.

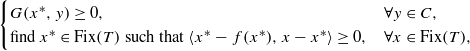We give an explicit Krasnoselski–Mann type method for finding common solutions of the following system of equilibrium and hierarchical fixed points: where C is a closed convex subset of a Hilbert space H, G:C×C→ℝ is an equilibrium function, T:C→C is a nonexpansive mapping with Fix(T) its set of fixed points and f:C→C is a ρ-contraction. Our algorithm is constructed and proved using the idea of the paper of [Y. Yao and Y.-C. Liou, ‘Weak and strong convergence of Krasnosel’skiĭ–Mann iteration for hierarchical fixed point problems’, Inverse Problems24 (2008), 501–508], in which only the variational inequality problem of finding hierarchically a fixed point of a nonexpansive mapping T with respect to a ρ-contraction f was considered. The paper follows the lines of research of corresponding results of Moudafi and Théra.

Keywords

hierarchical fixed pointsequilibrium problemnonexpansive mapcontractionvariational inequality problemprojection

MSC classification

Secondary: 47H09: Contraction-type mappings, nonexpansive mappings, $A$-proper mappings, etc. 47H10: Fixed-point theorems
Type
Research Article
Information
Bulletin of the Australian Mathematical Society , Volume 79 , Issue 2 , April 2009 , pp. 187 - 200
Copyright
Copyright © Australian Mathematical Society 2009

Footnotes

Supported by Ministero dell’Università e della Ricerca of Italy.

References

[1] Blum, E. and Oettli, E., ‘From optimation and variational inequalities to equilibrium problems’, Math. Student 63 (1994), 123–145.Google Scholar
[2] Browder, F. E., ‘Convergence of approximation to fixed points of nonexpansive nonlinear mappings in Hilbert spaces’, Arch. Ration. Mech. Anal. 24 (1967), 82–90.CrossRefGoogle Scholar
[3] Byrne, C., ‘A unified treatment of some iterative algorithms in signal processing and image reconstruction’, Inverse Problems 20 (2004), 103–120.CrossRefGoogle Scholar
[4] Cabot, A., ‘Proximal point algorithm controlled by a slowly vanishing term: applications to hierarchical minimization’, SIAM J. Optim. 15 (2005), 555–572.CrossRefGoogle Scholar
[5] Censor, Y., Motova, A. and Segal, A., ‘Perturbed projections and subgradient projections for the multiple-sets split feasibility problem’, J. Math. Anal. Appl. 327 (2007), 1244–1256.CrossRefGoogle Scholar
[6] Combettes, P. L. and Hirstoaga, S. A., ‘Equilibrium programming in Hilbert spaces’, J. Nonlinear Convex Anal. 6(1) (2005), 117–136.Google Scholar
[7] Flåm, S. D. and Antipin, A. S., ‘Equilibrium programming using proximal-like algorithms’, Math. Program. 78 (1997), 29–41.CrossRefGoogle Scholar
[8] Göpfert, A., Riahi, H., Tammer, C. and Zălinescu, C., Variational Methods in Partially Ordered Spaces (Springer, New York, 2003).Google Scholar
[9] Halpern, B., ‘Fixed points of nonexpansive maps’, Bull. Amer. Math. Soc. 73 (1967), 957–961.CrossRefGoogle Scholar
[10] Iusem, A. N. and Sosa, W., ‘Iterative algorithms for equilibrium problems’, Optimization 52 (2003), 301–316.CrossRefGoogle Scholar
[11] Konnov, I. V., ‘Application of the proximal point method to nonmonotone equilibrium problems’, J. Optim. Theory Appl. 119 (2003), 317–333.CrossRefGoogle Scholar
[12] Lions, P. L., ‘Approximation de points fixes de contractions’, C. R. Acad. Sci. Sér. A-B Paris 284 (1977), 1357–1359.Google Scholar
[13] Luo, Z.-Q., Pang, J.-S. and Ralph, D., Mathematical Programs with Equilibrium Constraints (Cambridge University Press, Cambridge, 1996).CrossRefGoogle Scholar
[14] Marino, G. and Xu, H. K., ‘A general iterative method for nonexpansive mappings in Hilbert spaces’, J. Math. Anal. Appl. 318(1) (2006), 43–52.CrossRefGoogle Scholar
[15] Moudafi, A., ‘Viscosity approximation methods for fixed-points problems’, J. Math. Anal. Appl. 241 (2000), 46–55.CrossRefGoogle Scholar
[16] Moudafi, A., ‘Second-order differential proximal methods for equilibrium problems’, J. Inequal. Pure Appl. Math. 4 (2003), art. 18.Google Scholar
[17] Moudafi, A., ‘Krasnoselski–Mann iteration for hierarchical fixed-point problems’, Inverse Problems 23 (2007), 1635–1640.CrossRefGoogle Scholar
[18] Moudafi, A. and Théra, M., ‘Proximal and dynamical approaches to equilibrium problems’, in: Ill-Posed Variational Problems and Regularization Techniques, Lecture Notes in Economics and Math. Sys., 477 (Springer, New York, 1999).Google Scholar
[19] Oettli, W., ‘A remark on vector-valued equilibria and generalized monotonicity’, Acta Math. Vietnamica 22 (1997), 215–221.Google Scholar
[20] Reich, S., ‘Strong convergence theorems for resolvents of accretive operators in Banach spaces’, J. Math. Anal. Appl. 75 (1980), 287–292.CrossRefGoogle Scholar
[21] Reich, S., ‘Approximating fixed points of nonexpansive mappings’, Panamerican. Math. J. 4(2) (1994), 23–28.Google Scholar
[22] Shioji, N. and Takahashi, W., ‘Strong convergence of approximated sequeces for nonexpansive mappings in Banach spaces’, Proc. Amer. Math. Soc. 125 (1997), 3641–3645.CrossRefGoogle Scholar
[23] Solodov, M., ‘An explicit descent method for bilevel convex optimization’, J. Convex Anal. 14 (2007), 227–237.Google Scholar
[24] Suzuki, T., ‘Strong convergence of Krasnoselskii and Mann’s type sequences for one-parameter nonexpansive semigroups without Bochner integrals’, J. Math. Anal. Appl. 305(1) (2005), 227–239.CrossRefGoogle Scholar
[25] Wittmann, R., ‘Approximation of fixed points of nonexpansive mappings’, Arch. Math. 58 (1992), 486–491.CrossRefGoogle Scholar
[26] Xu, H. K., ‘Iterative algorithms for nonlinear operators’, J. London Math. Soc. 66 (2002), 240–256.CrossRefGoogle Scholar
[27] Xu, H. K., ‘Another control condition in an iterative method for nonexpansive mappings’, Bull. Austral. Math. Soc. 65 (2002), 109–113.CrossRefGoogle Scholar
[28] Xu, H. K., ‘Remarks on an iterative method for nonexpansive mappings’, Comm. Appl. Nonlinear Anal. 10(1) (2003), 67–75.Google Scholar
[29] Xu, H. K., ‘An iterative approach to quadratic optimization’, J. Optim. Theory Appl. 116(3) (2003), 659–678.CrossRefGoogle Scholar
[30] Xu, H. K., ‘Viscosity approximation methods for nonexpansive mappings’, J. Math. Anal. Appl. 298 (2004), 279–291.CrossRefGoogle Scholar
[31] Xu, H. K., ‘A variable Krasnoselski–Mann algorithm and the multiple-set split feasibility problem’, Inverse Problems 22 (2006), 2021–2034.CrossRefGoogle Scholar
[32] Yamada, I., ‘The hybrid steepest descent method for the variational inequality problem over the intersection of fixed point sets of nonexpansive mappings’, in: Inherently Parallel Algorithm for Feasibility and Optimization and their Applications (eds. D. Butnariu, Y. Censor and S. Reich) (Elsevier, 2001), pp. 473–504.Google Scholar
[33] Yamada, I., Ogura, N. and Shirakawa, N., ‘A numerically robust hybrid steepest descent method for the convexity constrained generalized inverse problems’, Contemp. Math. 313 (2002), 269–305.CrossRefGoogle Scholar
[34] Yang, Q. and Zhao, J., ‘Generalized KM theorems and their applications’, Inverse Problems 22 (2006), 833–844.CrossRefGoogle Scholar
[35] Yao, Y. and Liou, Y.-C., ‘Weak and strong convergence of Krasnosel’skiĭ–Mann iteration for hierarchical fixed point problems’, Inverse Problems 24 (2008), 501–508.CrossRefGoogle Scholar