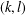Hostname: page-component-78c5997874-v9fdk Total loading time: 0 Render date: 2024-11-10T13:45:16.940Z Has data issue: false hasContentIssue false
  • English
  • Français

THE MAXIMUM SIZE OF $(k,l)$-SUM-FREE SETS IN CYCLIC GROUPS

Part of: Sequences and sets Additive number theory; partitions Extremal combinatorics Abelian groups

Published online by Cambridge University Press:  26 December 2018

BÉLA BAJNOK Open the ORCID record for BÉLA BAJNOK [Opens in a new window]  and
RYAN MATZKE Open the ORCID record for RYAN MATZKE [Opens in a new window]
BÉLA BAJNOK*
Affiliation:
Department of Mathematics, Gettysburg College, Gettysburg, PA, USA email bbajnok@gettysburg.edu
RYAN MATZKE
Affiliation:
School of Mathematics, University of Minnesota, Minneapolis, MN, USA email matzk053@umn.edu
*
✉bbajnok@gettysburg.edu
Rights & Permissions [Opens in a new window]

Abstract

Core share and HTML view are not available for this content. However, as you have access to this content, a full PDF is available via the ‘Save PDF’ action button.

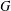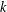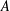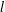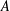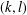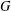A subset $A$ of a finite abelian group $G$ is called $(k,l)$-sum-free if the sum of $k$ (not necessarily distinct) elements of $A$ never equals the sum of $l$ (not necessarily distinct) elements of $A$. We find an explicit formula for the maximum size of a $(k,l)$-sum-free subset in $G$ for all $k$ and $l$ in the case when $G$ is cyclic by proving that it suffices to consider 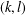$(k,l)$-sum-free intervals in subgroups of 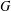$G$. This simplifies and extends earlier results by Hamidoune and Plagne [‘A new critical pair theorem applied to sum-free sets in abelian groups’, Comment. Math. Helv. 79(1) (2004), 183–207] and Bajnok [‘On the maximum size of a 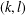$(k,l)$-sum-free subset of an abelian group’, Int. J. Number Theory 5(6) (2009), 953–971].

Keywords

sum-free setsabelian groupsKneser’s theoremarithmetic progressions

MSC classification

Primary: 11B75: Other combinatorial number theory
Secondary: 05D99: None of the above, but in this section 11B25: Arithmetic progressions 11P70: Inverse problems of additive number theory, including sumsets 20K01: Finite abelian groups
Type
Research Article
Information
Bulletin of the Australian Mathematical Society , Volume 99 , Issue 2 , April 2019 , pp. 184 - 194
Copyright
© 2018 Australian Mathematical Publishing Association Inc. 

References

Bajnok, B., ‘On the maximum size of a (k, l)-sum-free subset of an abelian group’, Int. J. Number Theory 5(6) (2009), 953–971.Google Scholar
Bajnok, B., Additive Combinatorics: A Menu of Research Problems (CRC Press, Boca Raton, FL, 2018).Google Scholar
Balasubramanian, R., Prakash, G. and Ramana, D. S., ‘Sum-free subsets of finite abelian groups of type III’, European J. Combin. 58 (2016), 181–202.Google Scholar
Bier, T. and Chin, A. Y. M., ‘On (k, l)-sets in cyclic groups of odd prime order’, Bull. Aust. Math. Soc. 63(1) (2001), 115–121.Google Scholar
Chervak, O., Pikhurko, O. and Staden, K., ‘Minimum number of additive tuples in groups of prime order’, Preprint, 2017, arXiv:1710.01936 [math.CO].Google Scholar
Diananda, P. H. and Yap, H. P., ‘Maximal sum-free sets of elements of finite groups’, Proc. Japan Acad. 45 (1969), 1–5.Google Scholar
Erdős, P., ‘Extremal problems in number theory’, in: Proc. Sympos. Pure Mathematics, Vol. VIII (American Mathematical Society, Providence, RI, 1965), 181–189.Google Scholar
Green, B. and Ruzsa, I., ‘Sum-free sets in abelian groups’, Israel J. Math. 147 (2005), 157–188.Google Scholar
Hamidoune, Y. O. and Plagne, A., ‘A new critical pair theorem applied to sum-free sets in abelian groups’, Comment. Math. Helv. 79(1) (2004), 183–207.Google Scholar
Plagne, A., ‘Maximal (k, l)-free sets in ℤ/pℤ are arithmetic progressions’, Bull. Aust. Math. Soc. 65(3) (2002), 137–144.Google Scholar
Samotij, W. and Sudakov, B., ‘The number of additive triples in subsets of abelian groups’, Math. Proc. Cambridge Philos. Soc. 160(3) (2016), 495–512.Google Scholar
Samotij, W. and Sudakov, B., ‘The number of additive triples in subsets of abelian groups’, Preprint, 2015, arXiv:1507.03764 [math.NT].Google Scholar
Street, A. P., ‘Maximal sum-free sets in abelian groups of order divisible by three’, Bull. Aust. Math. Soc. 6 (1972), 439–441.Google Scholar
Street, A. P., ‘Corrigendum: “Maximal sum-free sets in abelian groups of order divisible by three”’, Bull. Aust. Math. Soc. 7 (1972), 317–318.Google Scholar
Wallis, W. D., Street, A. P. and Wallis, J. S., Combinatorics: Room Squares, Sum-free Sets, Hadamard Matrices, Lecture Notes in Mathematics, 292 (Springer, Berlin–New York, 1972).Google Scholar
Yap, H. P., ‘Maximal sum-free sets in finite abelian groups. II’, Bull. Aust. Math. Soc. 5 (1971), 43–54.Google Scholar