No CrossRef data available.
Published online by Cambridge University Press: 17 April 2009
The object of this paper is to investigate under very general conditions the existence and mean-square stability of a random solution of a class of stochastic integral equations in the form
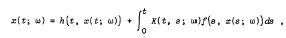
for t ≥ 0, where a random solution is a second order stochastic process {x(t; w) t ≥ 0} which satisfies the equation almost certainly. A random solution x(t; w) is defined to be stable in mean-square if E[|x(t; w)|2] ≤ p for all t ≥ 0 and some p > 0 or exponentially stable in mean-square if E[|x(t; w)|2] ≤ pe-at, t ≥ 0, for some constants ρ > 0 and α > 0.