Article contents
THE MULTIPLIER ALGEBRA OF A BEURLING ALGEBRA
Published online by Cambridge University Press: 15 May 2014
Abstract
For a discrete abelian cancellative semigroup  $\def \xmlpi #1{}\def \mathsfbi #1{\boldsymbol {\mathsf {#1}}}\let \le =\leqslant \let \leq =\leqslant \let \ge =\geqslant \let \geq =\geqslant \def \Pr {\mathit {Pr}}\def \Fr {\mathit {Fr}}\def \Rey {\mathit {Re}}S$ with a weight function
$\def \xmlpi #1{}\def \mathsfbi #1{\boldsymbol {\mathsf {#1}}}\let \le =\leqslant \let \leq =\leqslant \let \ge =\geqslant \let \geq =\geqslant \def \Pr {\mathit {Pr}}\def \Fr {\mathit {Fr}}\def \Rey {\mathit {Re}}S$ with a weight function 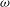 $\omega $ and associated multiplier semigroup
$\omega $ and associated multiplier semigroup 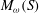 $M_\omega (S)$ consisting of
$M_\omega (S)$ consisting of 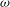 $\omega $-bounded multipliers, the multiplier algebra of the Beurling algebra of
$\omega $-bounded multipliers, the multiplier algebra of the Beurling algebra of 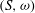 $(S,\omega )$ coincides with the Beurling algebra of
$(S,\omega )$ coincides with the Beurling algebra of 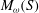 $M_\omega (S)$ with the induced weight.
$M_\omega (S)$ with the induced weight.
MSC classification
- Type
- Research Article
- Information
- Copyright
- Copyright © 2014 Australian Mathematical Publishing Association Inc.
References
- 4
- Cited by


