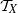Crossref Citations
This article has been cited by the following publications. This list is generated based on data provided by Crossref.
Sun, Lei
2024.
Green’s relations on the variant semigroups of all transformations of a set that reflect an equivalence.
Semigroup Forum,
Vol. 108,
Issue. 3,
p.
724.