A NOTE ON NORMALISED GROUND STATES FOR THE TWO-DIMENSIONAL CUBIC-QUINTIC NONLINEAR SCHRÖDINGER EQUATION

Abstract

We consider the two-dimensional minimisation problem for $\inf \{ E_a(\varphi ):\varphi \in H^1(\mathbb {R}^2)\ \text {and}\ \|\varphi \|_2^2=1\}$, where the energy functional 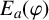$ E_a(\varphi )$ is a cubic-quintic Schrödinger functional defined by 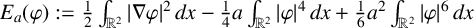$E_a(\varphi ):=\tfrac 12\int _{\mathbb {R}^2}|\nabla \varphi |^2\,dx-\tfrac 14a\int _{\mathbb {R}^2}|\varphi |^4\,dx+\tfrac 16a^2\int _{\mathbb {R}^2}|\varphi |^6\,dx$. We study the existence and asymptotic behaviour of the ground state. The ground state 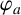$\varphi _{a}$ exists if and only if the 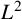$L^2$ mass a satisfies 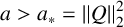$a>a_*={\lVert Q\rVert }^2_2$, where Q is the unique positive radial solution of 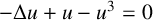$-\Delta u+ u-u^3=0$ in 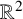$\mathbb {R}^2$. We show the optimal vanishing rate 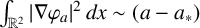$\int _{\mathbb {R}^2}|\nabla \varphi _{a}|^2\,dx\sim (a-a_*)$ as $a\searrow a_*$ and obtain the limit profile.

1 Introduction and main results

We consider the two-dimensional (2D) cubic-quintic nonlinear Schrödinger equation

(1.1) $$ \begin{align} i\psi_t=-\Delta\psi-|\psi|^2\psi+|\psi|^{4}\psi,\quad(t,x)\in\mathbb{R}\times\mathbb{R}^2, \end{align} $$

where the cubic nonlinearity is known as the Kerr nonlinearity [4] and the quintic nonlinearity was introduced in [15]. The incorporation of the defocusing quintic term is motivated by the stabilisation of two-dimensional vortex solitons [13]. This kind of model can be used to describe nonlinear optics, field theory, the mean-field theory of superconductivity, the motion of Bose–Einstein condensates and Langmuir waves in plasma physics (see [4] and the references therein).

The combination of a focusing cubic nonlinearity and defocusing quintic nonlinearity is very natural in many physical applications and leads to interesting mathematics. The nonlinear Schrödinger equations with the cubic-quintic nonlinearity (or general combined power-type nonlinearities) is very different from the purely cubic equation, since an effect of the quintic term is to prevent finite time blow-up (see [2]). Moreover, the arguments on the asymptotic behaviour of minimisers become much more complex and some new phenomena appear.

In particular, Soave [16, 17] studied normalised ground states for the nonlinear Schrödinger equation with combined nonlinearities. The uniqueness and nondegeneracy of positive solutions for the time-independent cubic-quintic nonlinear Schrödinger equation was shown in [1, 11]. Tao et al. [18] considered the Schrödinger equation with combined power-type nonlinearities including the cubic-quintic nonlinearity and studied local and global well-posedness, scattering, finite time blow-up and asymptotic behaviour. Killip et al. [9, 10] studied solitons, scattering and the initial-value problem with nonvanishing boundary conditions for the cubic-quintic nonlinear Schrödinger equation on $\mathbb {R}^3$ .

We focus on the normalised ground states of (1.1) and define the energy functional

$$ \begin{align*} E(u):=\frac{1}{2}\int_{\mathbb{R}^2}|\nabla u|^2\,dx-\frac{1}{4}\int_{\mathbb{R}^2}|u|^4\,dx+\frac{1}{6}\int_{\mathbb{R}^2}|u|^6\,dx. \end{align*} $$

A standing wave is a solution of (1.1) of the form

$$ \begin{align*}\psi(x,t)=e^{-i\lambda t}u(x),\end{align*} $$

where $\lambda \in \mathbb {R}$ and $u(x)\in H^1(\mathbb {R}^2)$ is a time-independent function. Usually, $u(x)$ is called a normalised ground state if it is a minimiser of the minimising problem under the prescribed $L^2$ mass:

$$ \begin{align*} I(a):=\inf\lbrace E(u):u\in H^1(\mathbb{R}^2) \text{ and } \|u\|_2^2=a\rbrace. \end{align*} $$

Let $\varphi (x)=u(x)/\sqrt {a}$ . It is easy to check that u is a minimiser of $I(a)$ if and only if $\varphi (x)$ is a minimiser of the minimisation problem for $e(a)$ , where $I(a)=a e(a)$ ,

(1.2) $$ \begin{align} e(a):=\inf\lbrace E_a(\varphi):\varphi\in H^1(\mathbb{R}^2) \text{ and } \|\varphi\|_2^2=1\rbrace, \end{align} $$

and the energy functional is given by

$$ \begin{align*} E_a(\varphi):=\frac{1}{2}\int_{\mathbb{R}^2}|\nabla \varphi|^2\,dx-\frac{a}{4}\int_{\mathbb{R}^2}|\varphi|^4\,dx+\frac{a^2}{6}\int_{\mathbb{R}^2}|\varphi|^6\,dx. \end{align*} $$

In what follows, we will consider this equivalent minimisation problem for $e(a)$ .

Now let

$$ \begin{align*} a_*:=\int_{\mathbb{R}^2}|Q|^2\,dx, \end{align*} $$

where Q is the unique positive radial solution of the nonlinear scalar field equation

(1.3) $$ \begin{align} -\Delta u+u-u^3=0,\quad u\in H^1(\mathbb{R}^2). \end{align} $$

The following theorem follows by the same arguments as in [1].

Theorem 1.1. Let Q be the unique positive radial solution of (1.3). Then:

1. (1) if $0< a\leq a_*={\lVert Q\rVert }^2_2$ , there is no minimiser for (1.2);

2. (2) if $a>a_*$ , there exists at least one minimiser for (1.2).

Moreover, $e(a)=0$ for $0< a\leq a_*$ and $\lim _{a\searrow a_*}e(a)=e(a_*)=0$ for $a>a_*$ .

Remark 1.2. We can restrict the minimiser of (1.2) to nonnegative radially symmetric functions, since $E_a(\varphi )\geq E_a(|\varphi |)$ for any $\varphi \in H^1(\mathbb {R}^2)$ (from the fact that $|\nabla |\varphi ||\leq |\nabla \varphi |$ almost everywhere (a.e.) in $\mathbb {R}^2$ ) and the symmetric decreasing rearrangement. Therefore, in what follows, we will assume that the ground state $\varphi _a(x)$ of (1.2) is nonnegative and radially symmetric decreasing.

In view of Theorem 1.1, it is natural to ask what would happen for minimisers $\varphi _a$ of $e(a)$ as $a\searrow a_*$ . We obtain the following result.

Theorem 1.3. Assume that $a>a_*$ and $\varphi _a$ is a nonnegative radially symmetric ground state of $e(a)$ . Then,

(1.4) $$ \begin{align} \lim_{a\searrow a_*}\int_{\mathbb{R}^2}|\nabla \varphi_a|^2\,dx\to 0\quad \text{and}\quad \int_{\mathbb{R}^2}|\nabla \varphi_a|^2\,dx\sim(a-a_*). \end{align} $$

Given a sequence $\{a_k\}$ with $a_k\searrow a_*$ as $k\to \infty $ , there exists a subsequence (still denoted by $\{a_k\}$ ) such that

(1.5) $$ \begin{align} (a_k-a_*)^{-{1}/{2}}\varphi_{a_k}( (a_k-a_*)^{-{1}/{2}}x) \to w_0(x) \quad \text{strongly in}\ H^1(\mathbb{R}^2), \end{align} $$

where $w_0$ satisfies

$$ \begin{align*}-\Delta w_0(x)=-\beta^2 w_0(x)+a_*w_0^3(x)-a_*^2w_0^5(x)\quad\text{for some } \beta \mbox{ with } 0<\beta^2<\tfrac{3}{16}.\end{align*} $$

Moreover,

(1.6) $$ \begin{align} \lim_{a\searrow a_*}(a-a_*)^{-{2}}e(a)=-\frac{a_*^2}{6}\int_{\mathbb{R}^2}|w_0|^6\,dx. \end{align} $$

Remark 1.4. From (1.4), the vanishing phenomenon happens for the ground states as $a\searrow a_*$ . This is very different from the purely cubic equation or the cubic-quintic equation with an external potential (see [3, 5–8, 14, 19]). In particular, Guo and Seiringer [5] studied the mass concentration properties of normalised ground-state solutions for the purely cubic equation with an external potential as $a\nearrow a_*$ ( $a<a_*$ and a tends to $a_*$ ). The second author and Feng [19] studied the blow-up properties of ground-state solutions of the 2D cubic-quintic nonlinear Schrödinger equation with a harmonic potential.

The paper is organised as follows. In Section 2, we prove Theorem 1.1. In Section 3, we prove Theorem 1.3. Throughout this paper, we use standard notation. For simplicity, we write $\|\cdot \|_p$ to denote the $L^p(\mathbb {R}^2)$ norm for $p\geq 1$ ; $a\searrow a_*$ means that a tends to $a_*$ with $a>a_*$ ; $X\sim Y$ means $X\lesssim Y$ and $Y\lesssim X$ , where $X\lesssim Y\ (X\gtrsim Y)$ means $X\leq CY\ (X\geq CY)$ for some appropriate positive constants C. The value of the positive constant C is allowed to change from line to line and also in the same formula.

2 Proof of Theorem 1.1

We recall from [20] that $ a_*$ also corresponds to the best constant in the Gagliardo–Nirenberg inequality

(2.1) $$ \begin{align} \int_{\mathbb{R}^2}|\varphi(x)|^4\,dx\leq\frac{2}{a_*}\int_{\mathbb{R}^2}|\nabla \varphi(x)|^2\,dx\int_{\mathbb{R}^2}|\varphi(x)|^2\,dx,\quad \varphi(x)\in H^1(\mathbb{R}^2), \end{align} $$

which becomes an equality when $\varphi (x) = Q(|x|)$ , where Q is the unique positive radial solution of (1.3). It is easy to see that

(2.2) $$ \begin{align} \frac{1}{2}\int_{\mathbb{R}^2}|Q(x)|^4\,dx=\int_{\mathbb{R}^2}|\nabla Q(x)|^2\,dx=\int_{\mathbb{R}^2}|Q(x)|^2\,dx \end{align} $$

(see also [2, Lemma 8.1.2]).

Lemma 2.1. For any $a>0$ , we have $e(a)\leq 0$ and $e(a)<0$ if and only if $a>a_*$ .

Proof. Let Q be the unique positive radial solution of (1.3). For $\gamma>0$ , define

$$ \begin{align*} \varphi_\gamma(x):=\frac{\gamma Q (\gamma x)}{\|Q\|_{2}}, \end{align*} $$

so that $\|\varphi _\gamma (x)\|^2_{2}=1$ . Since $\|\nabla Q\|^2_{2}=\tfrac 12\|Q\|^4_{4}=a_*$ (by (2.2)), then,

(2.3) $$ \begin{align} E_a(\varphi_\gamma)&=\frac{1}{2}\int_{\mathbb{R}^2}|\nabla \varphi_\gamma|^2\,dx-\frac{a}{4}\int_{\mathbb{R}^2}|\varphi_\gamma|^4\,dx+\frac{a^2}{6}\int_{\mathbb{R}^2}|\varphi_\gamma|^6\,dx\nonumber\\ &=\frac{\gamma^2}{2}\bigg(1-\frac{a}{a_*} \bigg)+\frac{a^2\gamma^{4}}{6a_*^3}\int_{\mathbb{R}^2}|Q|^6\,dx. \end{align} $$

By letting $\gamma \to 0^+$ , we deduce that $e(a)\leq 0$ .

To prove that $e(a)=0$ if and only if $0<a\leq a_*$ , we just need to show that for ${0<a\leq a_*}$ , we have $E_a(\varphi )\geq 0$ for any $\varphi \in H^1(\mathbb {R}^2)$ . We deduce from the Gagliardo– Nirenberg inequality (2.1) that

$$ \begin{gather*} \begin{aligned} E_a(\varphi)=\frac{1}{2}\int_{\mathbb{R}^2}|\nabla\varphi|^2\,dx-\frac{a}{4}\int_{\mathbb{R}^2}|\varphi|^4\,dx+\frac{a^2}{6}\int_{\mathbb{R}^2}|\varphi|^6\,dx \geq\frac{a^2}{6}\int_{\mathbb{R}^2}|\varphi|^6\,dx\geq0. \end{aligned} \end{gather*} $$

Thus, $e(a)=0$ if and only if $0<a\leq a_*$ .

Next, we claim that for any $a>a_*$ , we have $e(a)<0$ . By (2.3),

$$ \begin{gather*} \begin{aligned} e(a)\leq\frac{\gamma^2}{2}\bigg(1-\frac{a}{a_*} \bigg)+\frac{a^2\gamma^{4}}{6a_*^3}\int_{\mathbb{R}^2}|Q|^6\,dx =:-A(a-a_*)\gamma^2+B\gamma^{4}, \end{aligned} \end{gather*} $$

where

$$ \begin{align*}A=\frac{1}{2a_*}>0\quad\text{and}\quad B=\frac{a^2}{6a_*^3}\int_{\mathbb{R}^2}|Q|^6\,dx>0.\end{align*} $$

Now, let $\gamma =C_0(a-a_*)^{1/2}$ , taking $C_0$ small enough so that $AC_0^2-BC_0^4> 0$ . Then,

(2.4) $$ \begin{align} e(a)\leq-( AC_0^2-BC_0^4)(a-a_*)^{2}\lesssim-(a-a_*)^{2}<0 \end{align} $$

for any $a>a_*$ . This completes the proof of the Lemma 2.1.

Proof of Theorem 1.1.

Part (2) of Theorem 1.1 comes from [1], or it can be proved by the standard concentration-compactness principle [12].

Next, we prove that there is no minimiser for (1.2) with $0<a\leq a_*=\|Q\|_{2}^2$ . Suppose that there exists a minimiser $\varphi _a$ with $0<a\leq a_*$ . As pointed out in Section 1, we can assume $\varphi _a$ to be nonnegative. We deduce from the Gagliardo–Nirenberg inequality (2.1) and $e(a)=0$ that

$$ \begin{align*} \frac{1}{2}\int_{\mathbb{R}^2}|\nabla \varphi_a|^2\,dx=\frac{a}{4} \int_{\mathbb{R}^2}|\varphi_a|^4\,dx \end{align*} $$

and

$$ \begin{align*} \int_{\mathbb{R}^2}|\varphi_a|^6\,dx=0 \end{align*} $$

for $0<a\leq a_*$ . This implies $\varphi _a=0$ a.e., which is a contradiction with $\| \varphi _a \|^2_2 =1$ . This completes the proof of the first part of Theorem 1.1.

To prove the stated properties of the energy $e(a)$ , note that Lemma 2.1 implies that $e(a)=0$ for $0<a\leq a_*=\|Q\|_{2}^2$ . We have already shown that $e(a)\lesssim -(a-a_*)^{2}$ for ${a>a_*}$ in (2.4), hence it remains to show that $\lim _{a\searrow a_*}e(a)=e(a_*)=0$ for $a>a_*$ . This will complete the proof of Theorem 1.1.

3 Asymptotic behaviour of ground states as $a\searrow a_*$

Suppose that $\varphi _a(x)$ is a ground state of $e(a)$ for $a>a_*$ . Then $\varphi _a(x)$ satisfies the Euler–Lagrange equation

(3.1) $$ \begin{align} -\Delta \varphi_a(x)=\lambda_a \varphi_a(x)+a\varphi_a^3(x)-a^2\varphi_a^5(x) \end{align} $$

for some suitable Lagrange multiplier $\lambda _a\in \mathbb {R}$ and the Pohozaev-type identity $\partial E_a(\tau \varphi _a(\tau x))|_{\tau =1}=0$ (see [2]), that is,

(3.2) $$ \begin{align} \int_{\mathbb{R}^2}|\nabla \varphi_a|^2\,dx-\frac{a}{2}\int_{\mathbb{R}^2}|\varphi_a|^4\,dx+\frac{2a^2}{3}\int_{\mathbb{R}^2}|\varphi_a|^6\,dx=0. \end{align} $$

Moreover, $\lambda _a$ in (3.1) can be given by

(3.3) $$ \begin{align} \lambda_a=-\frac{1}{2}\int_{\mathbb{R}^2}|\nabla \varphi_a|^2\,dx-\frac{a}{4}\int_{\mathbb{R}^2}|\varphi_a|^4\,dx. \end{align} $$

Lemma 3.1. For any $a>a_*$ , we have $e(a)\sim -(a-a_*)^2$ .

Proof. In view of (2.4), we just need to prove the lower bound. First, for any ${\varphi \in H^1(\mathbb {R}^2)}$ , by using the Hölder’s inequality and Young’s inequality with $\epsilon $ ,

(3.4) $$ \begin{align} \int_{\mathbb{R}^2}|\varphi|^4\,dx&\leq\bigg(\int_{\mathbb{R}^2}|\varphi|^2\,dx \bigg)^{{1}/{2}} \bigg( \int_{\mathbb{R}^2}|\varphi|^6\,dx\bigg)^{{1}/{2}}\nonumber\\ &\leq \frac{3(a-a_*)}{8a^2}\int_{\mathbb{R}^2}|\varphi|^2\,dx+ \frac{2a^2(a-a_*)^{-1}}{3}\int_{\mathbb{R}^2}|\varphi|^6\,dx. \end{align} $$

Then, for any $\varphi (x)\in H^1(\mathbb {R}^2)$ with $\|\varphi \|^2_{2}=1$ , by using the Gagliardo–Nirenberg inequality (2.1) and (3.4),

$$ \begin{gather*} \begin{aligned} E_a(\varphi) &=\frac{1}{2}\int_{\mathbb{R}^2}|\nabla \varphi|^2\,dx-\frac{a}{4}\int_{\mathbb{R}^2}|\varphi|^4\,dx+\frac{a^2}{6}\int_{\mathbb{R}^2}|\varphi|^6\,dx\\ &\geq-\frac{a-a_*}{4}\int_{\mathbb{R}^2}|\varphi|^4\,dx+\frac{a^2}{6}\int_{\mathbb{R}^2}|\varphi|^6\,dx\\ &\gtrsim-(a-a_*)^{2}. \end{aligned} \end{gather*} $$

This completes the proof of Lemma 3.1.

Lemma 3.2. Assume that $\varphi _a(x)$ is a ground state of $e(a)$ . Then for any $a>a_*$ ,

(3.5) $$ \begin{align} \int_{\mathbb{R}^2}|\nabla \varphi_a(x)|^2\,dx\sim\int_{\mathbb{R}^2}|\varphi_a(x)|^4\,dx \sim(a-a_*). \end{align} $$

Proof. From the Gagliardo–Nirenberg inequality (2.1),

(3.6) $$ \begin{align} \frac{1}{2}\int_{\mathbb{R}^2}|\nabla \varphi_a(x)|^2\,dx-\frac{a}{4}\int_{\mathbb{R}^2}|\varphi_a(x)|^4\,dx\geq-\frac{a-a_*}{4}\int_{\mathbb{R}^2}|\varphi_a(x)|^4\,dx. \end{align} $$

However, by the definition of $e(a)$ and Lemma 3.1,

(3.7) $$ \begin{align} \frac{1}{2}\int_{\mathbb{R}^2}|\nabla \varphi_a(x)|^2\,dx-\frac{a}{4}\int_{\mathbb{R}^2}|\varphi_a(x)|^4\,dx\leq E_a(\varphi_a)=e(a)\lesssim-(a-a_*)^2. \end{align} $$

From the inequalities (3.6) and (3.7),

$$ \begin{gather*} \begin{aligned} \int_{\mathbb{R}^2}|\varphi_a(x)|^4\,dx\gtrsim(a-a_*). \end{aligned} \end{gather*} $$

Moreover, by the Gagliardo–Nirenberg inequality (2.1) and (3.7),

$$ \begin{align*} \int_{\mathbb{R}^2}|\nabla \varphi_a(x)|^2\,dx\gtrsim\int_{\mathbb{R}^2}|\varphi_a(x)|^4\,dx\gtrsim(a-a_*). \end{align*} $$

From (3.1), (3.2) and Lemma 3.1,

(3.8) $$ \begin{align} \frac{a^2}{6}\int_{\mathbb{R}^2}|\varphi_a|^6\,dx=-e(a)\sim(a-a_*)^2. \end{align} $$

By Hölder’s inequality together with (3.7) and (3.8),

$$ \begin{gather*} \begin{aligned} \int_{\mathbb{R}^2}|\nabla \varphi_a(x)|^2\,dx\lesssim\int_{\mathbb{R}^2}|\varphi_a(x)|^4\,dx\lesssim\bigg( \int_{\mathbb{R}^2}|\varphi_a|^6\,dx\bigg)^{{1}/{2}} \lesssim(a-a_*). \end{aligned} \end{gather*} $$

This completes the proof of the lemma.

Let $\varphi _a$ be a nonnegative minimiser of (1.2) and define the $L^2$ -normalised function

$$ \begin{align*} w_\tau(x):=\tau \varphi_a(\tau x), \end{align*} $$

where $\tau :=(a-a_*)^{-{1}/{2}}>0$ . From (3.5) and (3.8),

(3.9) $$ \begin{align} \int_{\mathbb{R}^2}|\nabla w_\tau(x)|^2\,dx\sim\int_{\mathbb{R}^2}|w_\tau(x)|^4\,dx\sim\int_{\mathbb{R}^2}|w_\tau(x)|^6\,dx\sim1. \end{align} $$

By the Euler–Lagrange equation (3.1) and Remark 1.2, the functions $w_\tau $ are nonnegative solutions and satisfy

(3.10) $$ \begin{align} -\Delta w_\tau(x)=\tau^2\lambda_a w_\tau(x)+aw_\tau^3(x)-a^2w_\tau^5(x). \end{align} $$

It follows from Lemma 3.2 and (3.3) that $\tau ^2\lambda _a$ is uniformly bounded as $a\searrow a_*$ and strictly negative for a close to $a_*$ . By passing to a subsequence, if necessary, we can thus assume that

(3.11) $$ \begin{align} \tau^2\lambda_a\to-\beta^2<0,\quad\text{as}\ a\searrow a_*. \end{align} $$

Proof of Theorem 1.3.

First, (1.4) in Theorem 1.3 comes from (3.5). Next, we prove (1.5). Note that $\{w_\tau \}$ is radially symmetric, since $\varphi _a$ is radially symmetric (see Remark 1.2). By (3.9), $\{w_\tau \}$ is uniformly bounded in $H_{\mathrm {rad}}^1(\mathbb {R}^2)$ and there exists a subsequence $\{w_{\tau _k}\}$ such that $w_{\tau _k}\rightharpoonup w_0$ weakly in $H_{{\mathrm { rad}}}^1(\mathbb {R}^2)$ , where $H_{{\mathrm {rad}}}^1( \mathbb {R}^2)$ denotes the Sobolev space of radial $H^1( \mathbb {R}^2)$ functions. For $2<p<+\infty $ , the embedding $H_{{\mathrm {rad}}}^1(\mathbb {R}^2)\hookrightarrow L^p(\mathbb {R}^2)$ is compact, so $w_{\tau _k}\to w_0$ strongly in $L^p(\mathbb {R}^2)$ . This implies that

(3.12) $$ \begin{align} \int_{\mathbb{R}^2}|w_{\tau_k}|^4\,dx\to\int_{\mathbb{R}^2} |w_0|^4\,dx\quad\text{and}\quad\int_{\mathbb{R}^2}|w_{\tau_k}|^6\,dx\to\int_{\mathbb{R}^2}|w_0|^6\,dx. \end{align} $$

By the Pohozaev identity (3.2), $w_{\tau _k}(x)$ satisfies

$$ \begin{gather*} \begin{aligned} \int_{\mathbb{R}^2}|\nabla w_{\tau_k}|^2\,dx-\frac{a_k}{2}\int_{\mathbb{R}^2}|w_{\tau_k}|^4\,dx+\frac{2a_k^2}{3}\int_{\mathbb{R}^2}|w_{\tau_k}|^6\,dx=0 \end{aligned} \end{gather*} $$

and it follows from (3.12) that

(3.13) $$ \begin{align} \lim_{k\to\infty}\int_{\mathbb{R}^2}|\nabla w_{\tau_k}|^2\,dx= \frac{a_*}{2}\int_{\mathbb{R}^2}|w_{0}|^4\,dx+\frac{2a_*^2}{3}\int_{\mathbb{R}^2}|w_{0}|^6\,dx. \end{align} $$

By passing to the weak limit $\tau _k\to 0^+$ in (3.10), we see that $w_0(x)$ satisfies

(3.14) $$ \begin{align} -\Delta w_0(x)=-\beta^2 w_0(x)+a_*w_0^3(x)-a_*^2w_0^5(x). \end{align} $$

We also have the Pohozaev identity (see [1]),

(3.15) $$ \begin{align} \beta^2\int_{\mathbb{R}^2}|w_0 |^2\,dx-\frac{a_*}{2}\int_{\mathbb{R}^2}|w_0 |^4\,dx+\frac{a_*^2}{3}\int_{\mathbb{R}^2}|w_0|^6\,dx=0, \end{align} $$

where $\beta ^2\in (0,3/16)$ since $w_0\not = 0$ (see [1]). From (3.14) and (3.15),

(3.16) $$ \begin{align} \int_{\mathbb{R}^2}|\nabla w_{0}|^2\,dx=\frac{a_*}{2}\int_{\mathbb{R}^2}|w_0|^4\,dx-\frac{2a_*^2}{3}\int_{\mathbb{R}^2}|w_0|^6\,dx. \end{align} $$

It follows from (3.13) that

(3.17) $$ \begin{align} \lim_{k\to\infty}\int_{\mathbb{R}^2}|\nabla w_{\tau_k}|^2\,dx=\int_{\mathbb{R}^2}|\nabla w_0|^2\,dx. \end{align} $$

However, by (3.11) together with (3.12) and (3.17),

$$ \begin{gather*} \begin{aligned} \lim_{k\to\infty} \int_{\mathbb{R}^2}|w_{\tau_k}|^2\,dx&=\lim_{k\to\infty}\frac{1}{\tau_k^2\lambda_{a_k}}\bigg( \int_{\mathbb{R}^2}|\nabla w_{\tau_k}|^2\,dx-a_k \int_{\mathbb{R}^2}|w_{\tau_k}|^4\,dx+ a_k^2\int_{\mathbb{R}^2}|w_{\tau_k}|^6\,dx\bigg) \\ &=-\frac{1}{\beta^2}\bigg( \int_{\mathbb{R}^2}|\nabla w_{0}|^2\,dx-a_* \int_{\mathbb{R}^2}|w_{0}|^4\,dx+a_*^2 \int_{\mathbb{R}^2}|w_{0}|^6\,dx\bigg)\\ &=\int_{\mathbb{R}^2}|w_0|^2\,dx. \end{aligned} \end{gather*} $$

Combining this with (3.17) shows $w_{\tau _k}\to w_0$ strongly in $H^1(\mathbb {R}^2)$ and this yields (1.5).

By (3.8) and (3.12),

(3.18) $$ \begin{align} \lim_{k\to\infty}\tau_k^4e(a_k)&=-\lim_{k\to\infty} \frac{\tau_k^4a_k^2}{6}\int_{\mathbb{R}^2}|\varphi_{a_k}|^6\,dx \nonumber\\ &=-\lim_{k\to\infty}\frac{a_k^2}{6}\int_{\mathbb{R}^2}|w_{\tau_k}|^6\,dx =-\frac{a_*^2}{6}\int_{\mathbb{R}^2}|w_{0}|^6\,dx. \end{align} $$

Finally, by applying the same argument as we used before to (3.18), we can take a subsequence $\{\tau _k\}$ with $\tau _k\to +\infty $ as $k\to +\infty $ , such that

(3.19) $$ \begin{align} \liminf_{a\searrow a_*}\tau^4e(a)=\lim_{k\to\infty}\tau_k^4e(a_k)=-\frac{a_*^2}{6}\int_{\mathbb{R}^2}|w_{0}|^6\,dx, \end{align} $$

where $w_0(x)$ satisfies (3.15) with some $\beta ^2\in (0,3/16)$ and $\int _{\mathbb {R}^2}|w_0(x)|^2\,dx=1$ . However, taking the test function $\phi _\tau =\tau ^{-1}w_0(\tau ^{-1}x)$ in $E_a(\cdot )$ , we deduce from (3.16) that

$$ \begin{gather*} \begin{aligned} \tau^4e(a)\leq \tau^4 E_a(\phi_\tau)&=\frac{1}{2}\int_{\mathbb{R}^2}|\nabla w_{0}|^2\,dx-\frac{a}{4}\int_{\mathbb{R}^2}|w_0|^4\,dx+\frac{a^2}{6}\int_{\mathbb{R}^2}|w_0|^6\,dx\\ &=\frac{a_*-a}{4}\int_{\mathbb{R}^2}|w_0|^4\,dx+\frac{a^2-2a_*^2}{6}\int_{\mathbb{R}^2}|w_0|^6\,dx. \end{aligned} \end{gather*} $$

This means that

$$ \begin{gather*} \begin{aligned} \limsup_{a\searrow a_*}\tau^4e(a)\leq-\frac{a_*^2}{6}\int_{\mathbb{R}^2}|w_{0}|^6\,dx. \end{aligned} \end{gather*} $$

Combining this with (3.19) gives (1.6). This completes the proof of Theorem 1.3.