Crossref Citations
This article has been cited by the following publications. This list is generated based on data provided by
Crossref.
ZHOU, SIZHONG
and
XU, YANG
2008.
NEIGHBOURHOODS OF INDEPENDENT SETS FOR (a,b,k)-CRITICAL GRAPHS.
Bulletin of the Australian Mathematical Society,
Vol. 77,
Issue. 2,
p.
277.
Zhou, Sizhong
2009.
Independence number, connectivity and (a,b,k)-critical graphs.
Discrete Mathematics,
Vol. 309,
Issue. 12,
p.
4144.
Liu, Hongxia
and
Liu, Guizhen
2009.
Binding number and minimum degree for the existence of (g,f,n)-critical graphs.
Journal of Applied Mathematics and Computing,
Vol. 29,
Issue. 1-2,
p.
207.
Liu, Shuli
2010.
On toughness and fractional -critical graphs.
Information Processing Letters,
Vol. 110,
Issue. 10,
p.
378.
ZHOU, SIZHONG
2010.
A SUFFICIENT CONDITION FOR A GRAPH TO BE A FRACTIONAL (f, n)-CRITICAL GRAPH.
Glasgow Mathematical Journal,
Vol. 52,
Issue. 2,
p.
409.
Zhou, Sizhong
2011.
Binding numbers and [a,b]-factors excluding a given k-factor.
Comptes Rendus. Mathématique,
Vol. 349,
Issue. 19-20,
p.
1021.
Zhou, Sizhong
and
Jiang, Jiashang
2011.
Toughness and -critical graphs.
Information Processing Letters,
Vol. 111,
Issue. 9,
p.
403.
Zhou, Sizhong
2011.
DEGREE CONDITIONS AND FRACTIONAL k-FACTORS OF GRAPHS.
Bulletin of the Korean Mathematical Society,
Vol. 48,
Issue. 2,
p.
353.
Zhou, Sizhong
Xu, Lan
and
Sun, Zhiren
2012.
Independence number and minimum degree for fractional ID-k-factor-critical graphs.
Aequationes mathematicae,
Vol. 84,
Issue. 1-2,
p.
71.
Zhou, Sizhong
Jiang, Jiashang
and
Xu, Lan
2012.
A binding number condition for graphs to be (a,b,k)-critical graphs.
Arab Journal of Mathematical Sciences,
Vol. 18,
Issue. 2,
p.
87.
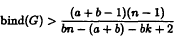 and
and
