Hostname: page-component-cd9895bd7-gbm5v Total loading time: 0 Render date: 2024-12-27T05:05:32.578Z Has data issue: false hasContentIssue false
  • English
  • Français

ON A PROBLEM ABOUT ADDITIVE REPRESENTATION FUNCTIONS

Part of: Sequences and sets

Published online by Cambridge University Press:  08 February 2022

MIN TANG Open the ORCID record for MIN TANG [Opens in a new window]
MIN TANG*
Affiliation:
School of Mathematics and Statistics, Anhui Normal University, Wuhu 241002, PR China
*
e-mail: tmzzz2000@163.com
Get access Rights & Permissions [Opens in a new window]

Abstract

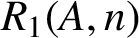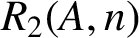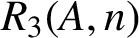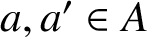For a set A of positive integers and any positive integer n, let $R_{1}(A, n)$ , $R_{2}(A,n)$ and $R_{3}(A,n)$ denote the number of solutions of $a+a^{\prime }=n$ with $a, a^{\prime }\in A$ and the additional restriction that 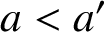$a<a^{\prime }$ for $R_{2}$ and 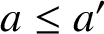$a\leq a^{\prime }$ for $R_{3}$ . We consider Problem 6 of Erdős et al. [‘On additive properties of general sequences’, Discrete Math. 136 (1994), 75–99] about locally small and locally large values of 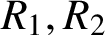$R_{1}, R_{2}$ and 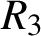$R_{3}$ .

Keywords

additive representation functionscharacteristic function

MSC classification

Primary: 11B13: Additive bases, including sumsets
Type
Research Article
Information
Bulletin of the Australian Mathematical Society , Volume 105 , Issue 3 , June 2022 , pp. 365 - 371
Check for updates
Copyright
© The Author(s), 2022. Published by Cambridge University Press on behalf of Australian Mathematical Publishing Association Inc.

Access options

Get access to the full version of this content by using one of the access options below. (Log in options will check for institutional or personal access. Content may require purchase if you do not have access.)

Footnotes

This work was supported by the National Natural Science Foundation of China (Grant No. 11971033) and top talents project of Anhui Department of Education (Grant No. gxbjZD05).

References

Balasubramanian, R., ‘A note on a result of Erdős, Sárközy and Sós’, Acta Arith. 49 (1987), 45–53.CrossRefGoogle Scholar
Balasubramanian, R. and Giri, S., ‘On additive representation functions’, Int. J. Number Theory 11 (2015), 1165–1176.CrossRefGoogle Scholar
Chen, Y. G., Sárközy, A., Sós, V. T. and Tang, M., ‘On the monotonicity properties of additive representation functions’, Bull. Aust. Math. Soc. 72 (2005), 129–138.CrossRefGoogle Scholar
Erdős, P. and Sárközy, A., ‘Problems and results on additive properties of general sequences I’, Pacific J. Math. 118 (1985), 347–357.CrossRefGoogle Scholar
Erdős, P. and Sárközy, A., ‘Problems and results on additive properties of general sequences II’, Acta Math. Hungar. 48 (1986), 201–211.CrossRefGoogle Scholar
Erdős, P., Sárközy, A. and Sós, V. T., ‘Problems and results on additive properties of general sequences III’, Studia Sci. Math. Hungar. 22 (1987), 53–63.Google Scholar
Erdős, P., Sárközy, A. and Sós, V. T., ‘Problems and results on additive properties of general sequences, IV’, in: Number Theory (Proceedings of the 4th Matscience Conference held at Ootacamund, India, January 5–10, 1984), Lecture Notes in Mathematics, 1122 (ed. Alladi, K.) (Springer-Verlag, Berlin, 1985), 85–104.Google Scholar
Erdős, P., Sárközy, A. and Sós, V. T., ‘Problems and results on additive properties of general sequences, V’, Monatsh. Math. 102 (1986), 183–197.CrossRefGoogle Scholar
Erdős, P., Sárközy, A. and Sós, V. T., ‘On additive properties of general sequences’, Discrete Math. 136 (1994), 75–99.CrossRefGoogle Scholar
Sárközy, A., ‘Unsolved problems in number theory’, Period. Math. Hungar. 42 (2001), 17–35.CrossRefGoogle Scholar
Sárközy, A., ‘On the number of additive representations of integers’, in: More Sets, Graphs and Numbers, Bolyai Society Mathematical Studies, 15 (eds. Győri, E., Katona, G. O. H. and Lovasz, L.) (Springer, Berlin, 2006), 329–339.CrossRefGoogle Scholar
Tang, M. and Chen, Y. G., ‘On the monotonicity properties of additive representation functions, II’, Discrete Math. 309 (2009), 1368–1373.CrossRefGoogle Scholar