No CrossRef data available.
Published online by Cambridge University Press: 17 April 2009
Suppose f is a function possessing a kth order derivative, the derivative being Lipschitz continuous of order α, 0 < α ≤ 1, on [−1, 1]. Let Pn be a polynomial of degree ≤ n approximating to f on [−1,1] such that if rn = f − Pn then ∥rn∥∞ ≤ An−k−a Define
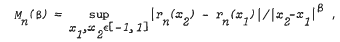
where 0 < β ≤ 1. Upper bounds are obtained for Mn(β) when k ≥ 1 thereby generalizing results previously given for functions which are only Lipschitz continuous on [−1,1].