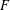Crossref Citations
This article has been cited by the following publications. This list is generated based on data provided by Crossref.
Jha, Abhishek
and
Sanna, Carlo
2022.
Greatest common divisors of shifted primes and Fibonacci numbers.
Research in Number Theory,
Vol. 8,
Issue. 4,
Jha, Abhishek
and
Sanna, Carlo
2023.
On the greatest common divisor ofnand thenth Fibonacci number, II.
Canadian Mathematical Bulletin,
Vol. 66,
Issue. 2,
p.
617.