Hostname: page-component-78c5997874-fbnjt Total loading time: 0 Render date: 2024-11-10T16:42:11.818Z Has data issue: false hasContentIssue false
  • English
  • Français

ON SUPERNILPOTENT NONSPECIAL RADICALS

Part of: Radicals and radical properties of rings

Published online by Cambridge University Press:  01 August 2008

HALINA FRANCE-JACKSON
HALINA FRANCE-JACKSON*
Affiliation:
Department of Mathematics and Applied Mathematics, Nelson Mandela Metropolitan University, Summerstrand Campus (South), PO Box 77000, Port Elizabeth 6031, South Africa (email: cbf@easterncape.co.uk)
Rights & Permissions [Opens in a new window]

Abstract

Core share and HTML view are not available for this content. However, as you have access to this content, a full PDF is available via the ‘Save PDF’ action button.

Let ρ be a supernilpotent radical. Let ρ* be the class of all rings A such that either A is a simple ring in ρ or the factor ring A/I is in ρ for every nonzero ideal I of A and every minimal ideal M of A is in ρ. Let 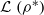be the lower radical determined by ρ* and let ρφ denote the upper radical determined by the class of all subdirectly irreducible rings with ρ-semisimple hearts. Le Roux and Heyman proved that 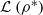is a supernilpotent radical with and they asked whether 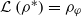if ρ is replaced by β, ℒ , 𝒩 or 𝒥 , where β, ℒ , 𝒩 and 𝒥 denote the Baer, the Levitzki, the Koethe and the Jacobson radical, respectively. In the present paper we will give a negative answer to this question by showing that if ρ is a supernilpotent radical whose semisimple class contains a nonzero nonsimple * -ring without minimal ideals, then 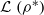is a nonspecial radical and consequently 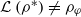. We recall that a prime ring A is a * -ring if A/I is in β for every .

Keywords

special radicalsupernilpotent radicalweakly special classspecial classprime essential ring*-ringsubdirectly irreducible ringessential ideal

MSC classification

Secondary: 16N80: General radicals and rings
Type
Research Article
Information
Bulletin of the Australian Mathematical Society , Volume 78 , Issue 1 , August 2008 , pp. 107 - 110
Copyright
Copyright © 2008 Australian Mathematical Society

References

[1]Divinsky, N., Rings and Radicals (Allen & Unwin, London, 1965).Google Scholar
[2]France-Jackson, H., ‘*-rings and their radicals’, Quaest. Math. 8(3) (1985), 231–239.CrossRefGoogle Scholar
[3]Gardner, B. J. and Stewart, P. N., ‘Prime essential rings’, Proc. Edinb. Math. Soc. 34 (1991), 241–250.CrossRefGoogle Scholar
[4]Gardner, B. J. and Wiegandt, R., Radical Theory of Rings (Marcel Dekker, New York, 2004).Google Scholar
[5]Le Roux, H. J. and Heyman, G. A. P., ‘A question on the characterization of certain upper radical classes’, Boll. Unione Mat. Ital. Sez. A 17(5) (1980), 67–72.Google Scholar