Article contents
ON THE LONGEST BLOCK FUNCTION IN CONTINUED FRACTIONS
Published online by Cambridge University Press: 13 February 2020
Abstract
For an irrational number 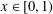 $x\in [0,1)$, let
$x\in [0,1)$, let  $x=[a_{1}(x),a_{2}(x),\ldots ]$ be its continued fraction expansion with partial quotients
$x=[a_{1}(x),a_{2}(x),\ldots ]$ be its continued fraction expansion with partial quotients 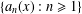 $\{a_{n}(x):n\geq 1\}$. Given
$\{a_{n}(x):n\geq 1\}$. Given  $\unicode[STIX]{x1D6E9}\in \mathbb{N}$, for
$\unicode[STIX]{x1D6E9}\in \mathbb{N}$, for 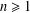 $n\geq 1$, the
$n\geq 1$, the  $n$th longest block function of
$n$th longest block function of  $x$ with respect to
$x$ with respect to 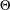 $\unicode[STIX]{x1D6E9}$ is defined by
$\unicode[STIX]{x1D6E9}$ is defined by 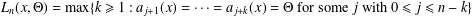 $L_{n}(x,\unicode[STIX]{x1D6E9})=\max \{k\geq 1:a_{j+1}(x)=\cdots =a_{j+k}(x)=\unicode[STIX]{x1D6E9}~\text{for some}~j~\text{with}~0\leq j\leq n-k\}$, which represents the length of the longest consecutive sequence whose elements are all
$L_{n}(x,\unicode[STIX]{x1D6E9})=\max \{k\geq 1:a_{j+1}(x)=\cdots =a_{j+k}(x)=\unicode[STIX]{x1D6E9}~\text{for some}~j~\text{with}~0\leq j\leq n-k\}$, which represents the length of the longest consecutive sequence whose elements are all 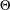 $\unicode[STIX]{x1D6E9}$ from the first
$\unicode[STIX]{x1D6E9}$ from the first  $n$ partial quotients of
$n$ partial quotients of  $x$. We consider the growth rate of
$x$. We consider the growth rate of 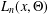 $L_{n}(x,\unicode[STIX]{x1D6E9})$ as
$L_{n}(x,\unicode[STIX]{x1D6E9})$ as 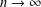 $n\rightarrow \infty$ and calculate the Hausdorff dimensions of the level sets and exceptional sets arising from the longest block function.
$n\rightarrow \infty$ and calculate the Hausdorff dimensions of the level sets and exceptional sets arising from the longest block function.
MSC classification
- Type
- Research Article
- Information
- Bulletin of the Australian Mathematical Society , Volume 102 , Issue 2 , October 2020 , pp. 196 - 206
- Copyright
- © 2020 Australian Mathematical Publishing Association Inc.
Footnotes
This work is supported by NSFC Grant No. 11431007.
References
- 3
- Cited by



