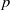Crossref Citations
This article has been cited by the following publications. This list is generated based on data provided by Crossref.
SU, NING
and
WANG, YANMING
2015.
ON THE -LENGTH AND THE WIELANDT SERIES OF A FINITE -SOLUBLE GROUP.
Bulletin of the Australian Mathematical Society,
Vol. 91,
Issue. 2,
p.
219.
Trofimuk, A.
2023.
On the $$p$$-length of a finite group with conditionally permutable factors.
Acta Mathematica Hungarica,
Vol. 170,
Issue. 1,
p.
405.