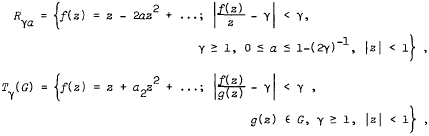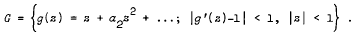Crossref Citations
This article has been cited by the following publications. This list is generated based on data provided by Crossref.
PORWAL, SAURABH
and
BULUT, SERAP
2015.
RADII PROBLEMS OF CERTAIN SUBCLASSES OF ANALYTIC FUNCTIONS WITH FIXED SECOND COEFFICIENTS.
Honam Mathematical Journal,
Vol. 37,
Issue. 3,
p.
317.
Verma, Shelly
and
Ravichandran, V.
2017.
Radius Problems for Ratios of Janowski Starlike Functions with Their Derivatives.
Bulletin of the Malaysian Mathematical Sciences Society,
Vol. 40,
Issue. 2,
p.
819.