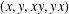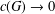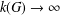Crossref Citations
This article has been cited by the following publications. This list is generated based on data provided by Crossref.
Martin-Pizarro, Amador
and
Palacín, Daniel
2024.
Complete type amalgamation for nonstandard finite groups.
Model Theory,
Vol. 3,
Issue. 1,
p.
1.