Article contents
TIGHT UNIVERSAL TRIANGULAR FORMS
Published online by Cambridge University Press: 18 November 2021
Abstract
For a subset S of nonnegative integers and a vector  $\mathbf {a}=(a_1,\ldots ,a_k)$ of positive integers, define the set
$\mathbf {a}=(a_1,\ldots ,a_k)$ of positive integers, define the set 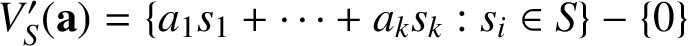 $V^{\prime }_S(\mathbf {a})=\{ a_1s_1+\cdots +a_ks_k : s_i\in S\}-\{0\}$. For a positive integer n, let
$V^{\prime }_S(\mathbf {a})=\{ a_1s_1+\cdots +a_ks_k : s_i\in S\}-\{0\}$. For a positive integer n, let 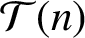 $\mathcal T(n)$ be the set of integers greater than or equal to n. We consider the problem of finding all vectors
$\mathcal T(n)$ be the set of integers greater than or equal to n. We consider the problem of finding all vectors 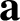 $\mathbf {a}$ satisfying
$\mathbf {a}$ satisfying 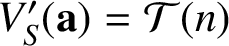 $V^{\prime }_S(\mathbf {a})=\mathcal T(n)$ when S is the set of (generalised) m-gonal numbers and n is a positive integer. In particular, we completely resolve the case when S is the set of triangular numbers.
$V^{\prime }_S(\mathbf {a})=\mathcal T(n)$ when S is the set of (generalised) m-gonal numbers and n is a positive integer. In particular, we completely resolve the case when S is the set of triangular numbers.
Keywords
Information
- Type
- Research Article
- Information
- Copyright
- © The Author(s), 2021. Published by Cambridge University Press on behalf of Australian Mathematical Publishing Association Inc.
Footnotes
This work was supported by a National Research Foundation of Korea (NRF) grant funded by the government of Korea (MSIT) (NRF-2021R1C1C2010133).
References
- 2
- Cited by


