No CrossRef data available.
Article contents
UNIQUENESS OF EXTENDABLE TEMPERATURES
Published online by Cambridge University Press: 02 October 2020
Abstract
Let E and D be open subsets of 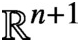 $\mathbb {R}^{n+1}$ such that
$\mathbb {R}^{n+1}$ such that 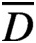 $\overline {D}$ is a compact subset of E, and let v be a supertemperature on E. A temperature u on D is called extendable by v if there is a supertemperature w on E such that
$\overline {D}$ is a compact subset of E, and let v be a supertemperature on E. A temperature u on D is called extendable by v if there is a supertemperature w on E such that 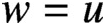 $w=u$ on D and
$w=u$ on D and 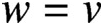 $w=v$ on
$w=v$ on  $E\backslash \overline D$. From earlier work of N. A. Watson, [‘Extendable temperatures’, Bull. Aust. Math. Soc. 100 (2019), 297–303], either there is a unique temperature extendable by v, or there are infinitely many; a necessary condition for uniqueness is that the generalised solution of the Dirichlet problem on D corresponding to the restriction of v to
$E\backslash \overline D$. From earlier work of N. A. Watson, [‘Extendable temperatures’, Bull. Aust. Math. Soc. 100 (2019), 297–303], either there is a unique temperature extendable by v, or there are infinitely many; a necessary condition for uniqueness is that the generalised solution of the Dirichlet problem on D corresponding to the restriction of v to 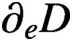 $\partial _eD$ is equal to the greatest thermic minorant of v on D. In this paper we first give a condition for nonuniqueness and an example to show that this necessary condition is not sufficient. We then give a uniqueness theorem involving the thermal and cothermal fine topologies and deduce a corollary involving only parabolic and coparabolic tusks.
$\partial _eD$ is equal to the greatest thermic minorant of v on D. In this paper we first give a condition for nonuniqueness and an example to show that this necessary condition is not sufficient. We then give a uniqueness theorem involving the thermal and cothermal fine topologies and deduce a corollary involving only parabolic and coparabolic tusks.
MSC classification
Information
- Type
- Research Article
- Information
- Copyright
- © 2020 Australian Mathematical Publishing Association Inc.


