Crossref Citations
This article has been cited by the following publications. This list is generated based on data provided by Crossref.
Fiorenza, Alberto
Gogatishvili, Amiran
and
Kopaliani, Tengiz
2013.
Boundedness of Stein’s spherical maximal function in variable Lebesgue spaces and application to the wave equation.
Archiv der Mathematik,
Vol. 100,
Issue. 5,
p.
465.
Lacey, Michael T.
2019.
Sparse bounds for spherical maximal functions.
Journal d'Analyse Mathématique,
Vol. 139,
Issue. 2,
p.
613.
Ho, Kwok-Pun
2019.
Spherical maximal functions, variation and oscillation inequalities on Herz spaces.
Arab Journal of Basic and Applied Sciences,
Vol. 26,
Issue. 1,
p.
72.
Roncal, Luz
Shrivastava, Saurabh
and
Shuin, Kalachand
2021.
Bilinear Spherical Maximal Functions of Product Type.
Journal of Fourier Analysis and Applications,
Vol. 27,
Issue. 4,
Ho, Kwok-Pun
2021.
Spherical maximal function, maximal Bochner–Riesz mean and geometrical maximal function on Herz spaces with variable exponents.
Rendiconti del Circolo Matematico di Palermo Series 2,
Vol. 70,
Issue. 1,
p.
559.
Manna, Ramesh
Shrivastava, Saurabh
and
Shuin, Kalachand
2021.
Weighted estimates for maximal functions associated with finite type curves in R2.
Nonlinear Analysis,
Vol. 205,
Issue. ,
p.
112225.
Deng, Chaohong
Sun, Jiawei
and
Li, Baode
2023.
Extrapolations on ball Banach function spaces and applications.
Annals of Functional Analysis,
Vol. 14,
Issue. 1,
Ho, Kwok-Pun
2024.
Rough Singular Integral Operators, Spherical Maximal Functions and Maximal Bochner-Riesz Operators on Grand Morrey Spaces.
Complex Analysis and Operator Theory,
Vol. 18,
Issue. 6,
Yee, Tat-Leung
Cheung, Ka Luen
Ho, Kwok-Pun
and
Suen, Chun Kit
2024.
Spherical Maximal Function on Local Morrey Spaces with Variable Exponents.
Vietnam Journal of Mathematics,
Vol. 52,
Issue. 1,
p.
107.
Hoang, Cong
Moen, Kabe
and
Pérez, Carlos
2024.
Pointwise estimates for rough operators with applications to Sobolev inequalities.
Journal d'Analyse Mathématique,
Ramadana, Yusuf
and
Gunawan, Hendra
2024.
Boundedness of sublinear operator generated by Calderón-Zygmund operator on generalized weighted Morrey spaces over quasi-metric measure spaces.
Vol. 3029,
Issue. ,
p.
040026.


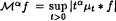 , where μ
, where μ