Published online by Cambridge University Press: 20 November 2018
For a positive finite measure 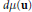 $d\mu \left( \mathbf{u} \right)$ on
$d\mu \left( \mathbf{u} \right)$ on 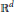 ${{\mathbb{R}}^{d}}$ normalized to satisfy
${{\mathbb{R}}^{d}}$ normalized to satisfy
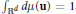 $\int{_{{{\mathbb{R}}^{d}}}d\mu \left( \mathbf{u} \right)}=1$
, the dilated average of
$\int{_{{{\mathbb{R}}^{d}}}d\mu \left( \mathbf{u} \right)}=1$
, the dilated average of 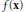 $f\left( \mathbf{x} \right)$ is given by
$f\left( \mathbf{x} \right)$ is given by
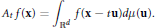 $${{A}_{t}}\,f\left( \mathbf{x} \right)\,=\,\int{_{{{\mathbb{R}}^{d}}}\,f\left( \mathbf{x}\,-\,t\mathbf{u}\, \right)}d\mu \left( \mathbf{u} \right)$$
$${{A}_{t}}\,f\left( \mathbf{x} \right)\,=\,\int{_{{{\mathbb{R}}^{d}}}\,f\left( \mathbf{x}\,-\,t\mathbf{u}\, \right)}d\mu \left( \mathbf{u} \right)$$
It will be shown that under some mild assumptions on 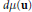 $d\mu \left( \mathbf{u} \right)$ one has the equivalence
$d\mu \left( \mathbf{u} \right)$ one has the equivalence
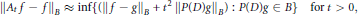 $$||{A_t}f - f|{|_B} \approx \inf \left\{ {\left( {||f - g|{|_B} + {t^2}||P\left( D \right)g|{|_B}} \right):P\left( D \right)g \in B} \right\}{\mkern 1mu} {\mkern 1mu} {\mkern 1mu} {\mkern 1mu} {\mkern 1mu} {\mkern 1mu} {\rm{for}}{\mkern 1mu} {\mkern 1mu} {\rm{t}}{\mkern 1mu} {\rm{ > }}\,{\rm{0,}}$$
$$||{A_t}f - f|{|_B} \approx \inf \left\{ {\left( {||f - g|{|_B} + {t^2}||P\left( D \right)g|{|_B}} \right):P\left( D \right)g \in B} \right\}{\mkern 1mu} {\mkern 1mu} {\mkern 1mu} {\mkern 1mu} {\mkern 1mu} {\mkern 1mu} {\rm{for}}{\mkern 1mu} {\mkern 1mu} {\rm{t}}{\mkern 1mu} {\rm{ > }}\,{\rm{0,}}$$
where
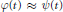 $\varphi \left( t \right)\approx \psi \left( t \right)$
means
$\varphi \left( t \right)\approx \psi \left( t \right)$
means
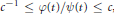 ${{c}^{-1}}\le \varphi \left( t \right)/\psi \left( t \right)\le c$
,
${{c}^{-1}}\le \varphi \left( t \right)/\psi \left( t \right)\le c$
, 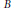 $B$ is a Banach space of functions for which translations are continuous isometries and
$B$ is a Banach space of functions for which translations are continuous isometries and 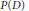 $P\left( D \right)$ is an elliptic differential operator induced by
$P\left( D \right)$ is an elliptic differential operator induced by 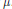 $\mu $. Many applications are given, notable among which is the averaging operator with
$\mu $. Many applications are given, notable among which is the averaging operator with
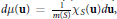 $d\mu \left( \mathbf{u} \right)\,=\,\frac{1}{m\left( S \right)}{{\chi }_{S}}\left( \mathbf{u} \right)d\mathbf{u},$
where
$d\mu \left( \mathbf{u} \right)\,=\,\frac{1}{m\left( S \right)}{{\chi }_{S}}\left( \mathbf{u} \right)d\mathbf{u},$
where  $S$ is a bounded convex set in
$S$ is a bounded convex set in 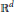 ${{\mathbb{R}}^{d}}$ with an interior point,
${{\mathbb{R}}^{d}}$ with an interior point, 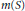 $m\left( S \right)$ is the Lebesgue measure of
$m\left( S \right)$ is the Lebesgue measure of  $S$, and
$S$, and 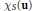 ${{\chi }_{S}}\left( \mathbf{u} \right)$ is the characteristic function of
${{\chi }_{S}}\left( \mathbf{u} \right)$ is the characteristic function of  $S$. The rate of approximation by averages on the boundary of a convex set under more restrictive conditions is also shown to be equivalent to an appropriate
$S$. The rate of approximation by averages on the boundary of a convex set under more restrictive conditions is also shown to be equivalent to an appropriate 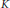 $K$-functional.
$K$-functional.