Hostname: page-component-cd9895bd7-gvvz8 Total loading time: 0 Render date: 2024-12-26T20:26:15.695Z Has data issue: false hasContentIssue false
  • English
  • Français

C*-Convexity and Matricial Ranges

Published online by Cambridge University Press:  20 November 2018

D. R. Farenick
D. R. Farenick*
Affiliation:
Centre de Recherches Mathématiques Université de Montréal Montréal, Québec H3C 3J7
Rights & Permissions [Opens in a new window]

Abstract

Core share and HTML view are not available for this content. However, as you have access to this content, a full PDF is available via the ‘Save PDF’ action button.

C* -convex sets in matrix algebras are convex sets of matrices in which matrix-valued convex coefficients are admitted along with the usual scalar-valued convex coefficients. A Carathéodory-type theorem is developed for C*-convex hulls of compact sets of matrices, and applications of this theorem are given to the theory of matricial ranges. If T is an element in a unital C*-algebra 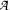, then for every n ∈ N, the n x n matricial range Wn(T) of T is a compact C* -convex set of n x n matrices. The basic relation W1(T) = conv σ-(T) is well known to hold if T exhibits the normal-like quality of having the spectral radius of β T + μ 1 coincide with the norm ||β T + μ 1|| for every pair of complex numbers β and μ. An extension of this relation to the matrix spaces is given by Theorem 2.6: Wn (T) is the C*-convex hull of the n x n matricial spectrum σn(T) of T if, for every B,M ∈ ℳn, the norm of T ⊗ B + 1 ⊗ M in 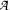⊗ ℳn is the maximum value in {||∧⊗B + 1 ⊗M|| : Λ ∈ σn (T)}. The spatial matricial range of a Hilbert space operator is the analogue of the classical numerical range, although it can fail to be convex if n > 1. It is shown in § 3 that if T has a normal dilation N with σ (N) ⊂ σ (T), then the closure of the spatial matricial range of T is convex if and only if it is C*-convex.

Keywords

Primary:47A12secondary:15A6046L05
Type
Research Article
Information
Canadian Journal of Mathematics , Volume 44 , Issue 2 , 01 April 1992 , pp. 280 - 297
Copyright
Copyright © Canadian Mathematical Society 1992

References

1. Arveson, W.B., Subalgebrasof C*-algebras, Acta Math. 123(1969), 141–224.Google Scholar
2. Arveson, W.B., Subalgebras of C*-algebras II, Acta Math. 129(1972), 271–308.Google Scholar
3. Azoff, E. and Ch. Davis, On distances between unitary orbits of self-adjoint operators, Acta Sci. Math. (Szeged) 47(1984), 419–439.Google Scholar
4. Bonsall, F. and Duncan, J., Numerical Ranges II. London Math. Soc. Lecture Note Series, no. 10, Cambridge University Press, Cambridge, 1972.Google Scholar
5. Bunce, J. and Salinas, N., Completely positive maps on C* -algebras and the left matricial spectrum of an operator, Duke Math. J. 43(1976), 747–774.Google Scholar
6. Davis, Ch., Beyond the minimaxprinciple, Proc. Amer. Math. Soc. 81(1981), 401–405.Google Scholar
7. Farenick, D.R., The matricial spectrum and range, and C*-convex sets. Dissertation, University of Toronto, 1990.Google Scholar
8. Hildebrandt, S., Überden numerischen Wertebereich eines Operators, Math. Ann. 163(1966), 230–247.Google Scholar
9. Li, C.-K., The C-convex matrices, Linear and Multilinear Algebra 21(1987), 249–257.Google Scholar
10. Li, C.-K. and Tsing, N.-K., On the kth matrix numerical range, Linear and Multilinear Algebra, 28(1991), 229–239.Google Scholar
11. Loebl, R.I. and Paulsen, V.I., Some remarks on C*-convexity, Linear Algebra Appl. 35(1981), 63–78.Google Scholar
12. Narcowich, F.J. and Ward, J.D., Support functions for matrix ranges: analogues of Lumer's formula, J. Operator Theory 7(1982), 25–49.Google Scholar
13. Paulsen, V.I., Completely bounded maps and dilations. Pitman Research Notes 146, Longman Scientific and Technical, New York, 1986.Google Scholar
14. Pearcy, C. and Salinas, N., The reducing essential matricial spectra of an operator, Duke Math. J. 42(1975), 423–434.Google Scholar
15. Pollack, F.M., Properties of the matrix range of an operator, Indiana Univ. Math. J. 22(1973), 419–427.Google Scholar
16. Poon, Y.-T., The generalized k-numerical range, Linear and Multilinear Algebra 9(1980), 181–186.Google Scholar
17. Salinas, N., Reducing essential eigenvalues, Duke Math. J. 40(1973), 561–580.Google Scholar
18. Salinas, N., Hypoconvexity and essentially n-normal operators, Trans. Amer. Math. Soc. 256(1979), 325–357.Google Scholar
19. Smith, R.R. and Ward, J.D., Matrix ranges for Hilbert space operators, Amer. J. Math. 102(1980), 1031 — 1081.Google Scholar
20. Stampfli, J.G. and Williams, J.P., Growth conditions and the numerical range in a Banach algebra, Tôhoku Math. J. 20(1968), 417–424.Google Scholar