Article contents
Characterizations of Continuous and Discrete q-Ultraspherical Polynomials
Published online by Cambridge University Press: 20 November 2018
Abstract
We characterize the continuous q-ultraspherical polynomials in terms of the special form of the coefficients in the expansion 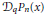 ${{\mathcal{D}}_{q}}{{P}_{n}}\left( x \right)$ in the basis
${{\mathcal{D}}_{q}}{{P}_{n}}\left( x \right)$ in the basis 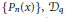 $\left\{ {{P}_{n}}\left( x \right) \right\},{{\mathcal{D}}_{q}}$ being the Askey-Wilson divided difference operator. The polynomials are assumed to be symmetric, and the connection coefficients are multiples of the reciprocal of the square of the
$\left\{ {{P}_{n}}\left( x \right) \right\},{{\mathcal{D}}_{q}}$ being the Askey-Wilson divided difference operator. The polynomials are assumed to be symmetric, and the connection coefficients are multiples of the reciprocal of the square of the 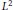 ${{L}^{2}}$ norm of the polynomials. A similar characterization is given for the discrete
${{L}^{2}}$ norm of the polynomials. A similar characterization is given for the discrete  $q$-ultraspherical polynomials. A new proof of the evaluation of the connection coefficients for big
$q$-ultraspherical polynomials. A new proof of the evaluation of the connection coefficients for big  $q$-Jacobi polynomials is given.
$q$-Jacobi polynomials is given.
Keywords
- Type
- Research Article
- Information
- Copyright
- Copyright © Canadian Mathematical Society 2011
References
- 1
- Cited by


