Hostname: page-component-78c5997874-ndw9j Total loading time: 0 Render date: 2024-11-15T15:28:13.600Z Has data issue: false hasContentIssue false
  • English
  • Français

Commutativity for Matrices of Quaternions

Published online by Cambridge University Press:  20 November 2018

R. E. Carlson  and
C. G. Cullen
R. E. Carlson
Affiliation:
University of Pittsburgh
C. G. Cullen
Affiliation:
University of Pittsburgh
Rights & Permissions [Opens in a new window]

Extract

Core share and HTML view are not available for this content. However, as you have access to this content, a full PDF is available via the ‘Save PDF’ action button.

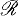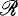For any ring we shall denote by the ring of all n × n matrices with elements from and by 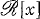the set of all polynomials in x with coefficients from .

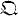will denote the non-commutative four-dimensional division algebra of real quaternions with 1, i1, i2, i3 as generators

Type
Research Article
Information
Canadian Journal of Mathematics , Volume 20 , 1968 , pp. 21 - 24
Copyright
Copyright © Canadian Mathematical Society 1968

Footnotes

Research supported by the National Science Foundation.

References

1. Cullen, C. G., Intrinsic functions on matrices of real quaternions, Can. J. Math., 15 (1963), 456–466.Google Scholar
2. Cullen, C. G., Matrices and linear transformations (Reading, Mass., 1966).Google Scholar
3. Rinehart, R. F., Elements of a theory of intrinsic functions on algebras, Duke Math. J., 27 1960), 1–19.Google Scholar
4. Rinehart, R. F., Intrinsic function on matrices, Duke Math. J., 28 (1961), 291–300.Google Scholar
5. Wiegman, N. A., Some theorems on matrices with real quaternion elements, Can. J. Math., 7 1955), 191–201.Google Scholar