Hostname: page-component-cd9895bd7-q99xh Total loading time: 0 Render date: 2024-12-28T13:18:03.808Z Has data issue: false hasContentIssue false
  • English
  • Français

Contributions to a General Theory of View-Obstruction Problems

Published online by Cambridge University Press:  20 November 2018

V. C. Dumir ,
R. J. Hans-Gill  and
J. B. Wilker
V. C. Dumir
Affiliation:
Centre for Advanced Study in Mathematics, Panjab University, Chandigarh 160014, India
R. J. Hans-Gill
Affiliation:
Centre for Advanced Study in Mathematics, Panjab University, Chandigarh 160014, India
J. B. Wilker
Affiliation:
Physical Sciences Division, Scarborough College, University of Toronto, West Hill, Ontario M1C 1A4
Rights & Permissions [Opens in a new window]

Abstract

Core share and HTML view are not available for this content. However, as you have access to this content, a full PDF is available via the ‘Save PDF’ action button.

In the original view-obstruction problem congruent closed, centrally symmetric convex bodies centred at the points of the set 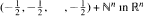are expanded uniformly until they block all rays from the origin into the open positive cone. The central problem is to determine the minimal blocking size and this value is known for balls in dimensions n = 2,3 and for symmetrically placed cubes in dimensions n = 2, 3, 4

In order to explain fully the distinction between rational and irrational rays in the original problem, we extend consideration to the blocking of subspaces of all dimensions. In order to appreciate the special properties of balls and cubes, we give a discussion of the convex body with respect to reflection symmetry, lower dimensional sections, and duality. We introduce topological considerations to help understand when the critical parameter of the theory is an attained maximum and we add substantially to the list of known values of this parameter. In particular, when the dimension is n = 2 our dual body considerations furnish a complete solution to the view-obstruction problem

Keywords

10E0552A30geometry of numbersview-obstruction problemKronecker's Theoremdual convex bodyBlaschke Selection Theorem
Type
Research Article
Information
Canadian Journal of Mathematics , Volume 45 , Issue 3 , 01 June 1993 , pp. 517 - 536
Copyright
Copyright © Canadian Mathematical Society 1993

References

1. Betke, U. and Wills, J. M., Untere Schranken fur zwei diophantische Approximations-Funktionen, Monat. fur Math. 76(1972), 214–217.Google Scholar
2. Cassels, J. W S., An Introduction to the Geometry of Numbers, Springer Verlag, Berlin, 1959.Google Scholar
3. Chen, Y. G., On a conjecture in Diophantine approximations, II, J. Number Theory, 37(1991), 181–198.Google Scholar
4. Chen, Y. G., On a conjecture in Diophantine approximations, III, J. Number Theory, 39(1991 ), 91–103.Google Scholar
5. Chen, Y. G., View-obstruction problem for 3-dimensional spheres, J. Number Theory, to appear.Google Scholar
6. Cusick, T. W., View-obstruction problems, Aequationes Math 9(1973), 165–170.Google Scholar
7. Cusick, T. W., View-obstruction problems in n-dimensional geometry, J. Combin. Theory, (A) 16(1974), 1–11.Google Scholar
8. Cusick, T. W., View-obstruction problems II, Proc. Amer. Math. Soc. 84(1982), 25–28.Google Scholar
9. Cusick, T. W. and Carl Pomerance, View-obstruction problems III, J. Number Theory 19(1984), 131–139.Google Scholar
10. Dumir, V. C. and Hans, R. J. Gill, View-obstruction problem for 3-dimensional spheres, Monat. fur Math. 101(1986), 279–290.Google Scholar
11. Dumir, V. C. and Hans, R. J. Gill, The view obstruction problem for boxes, J. Indian Math. Soc, 57(1991), 117–122.Google Scholar
12. Dumir, V. C. and Hans, R. J. Gill, Markofftype chain for the view-obstruction problem for three-dimensional cubes, J. Indian Math. Soc, 57(1991), 123–141.Google Scholar
13. Eggleston, H. G., Convexity, Cambridge University Press, 1969.Google Scholar
14. Hardy, G. H. and Wright, E. M., Introduction to the Theory of Numbers, 4th éd., 1959.Google Scholar
15. Lekkerkerker, C. G., Geometry of Numbers, North-Holland, Amsterdam, 1969.Google Scholar
16. Wills, J. M., Zur simultanen homogenen diophantischen Approximation I, Monat. fur Math. 72(1968), 254–263.Google Scholar