No CrossRef data available.
Published online by Cambridge University Press: 20 November 2018
Convergence of exponential sums
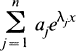
that interpolate to Laplace transforms
(1.1)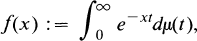
have been studied by several authors [3, 6, 8, 15]. For rational functions that interpolate to Markov functions (also called Hamburger or Stieltjes Series or Hilbert Transforms)
(1.2)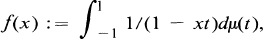
far more detailed convergence results are available (see [10, 11, 16] and references therein). Both (1.1) and (1.2) are special cases of the transform
(1.3)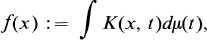
where K(x, t) is a strictly totally positive kernel.